Advertisements
Advertisements
Question
In the given figure, AB is the diameter of the circle, with centre O, and AT is the tangent. Calculate the numerical value of x.
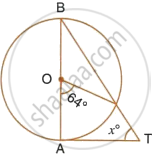
Solution
In ΔOBC,
OB = OC ...(Radii of the same circle)
∴ ∠OBC = ∠OCB
But, Ext. ∠COA = ∠OBC + ∠OCB
Ext. ∠COA = 2∠OBC
`=>` 64° = 2∠OBC
∴ ∠OBC = `64^circ/2` = 32°
Now in ΔABT,
∠BAT = 90° ...(OA ⊥ AT)
∠OBC or ∠ABT = 32°
∴ ∠BAT + ∠ABT + x° = 180°
`=>` 90° + 32° + x° = 180°
`=>` 122° + x° = 180°
`=>` x° = 180° – 122°
`=>` x° = 58°
APPEARS IN
RELATED QUESTIONS
Two circle touch each other externally at point P. Q is a point on the common tangent through P. Prove that the tangents QA and QB are equal.

In a triangle ABC, the incircle (centre O) touches BC, CA and AB at points P, Q and R respectively. Calculate:
i)`∠`QPR .
AB is the diameter and AC is a chord of a circle with centre O such that angle BAC = 30°. The tangent to the circle at C intersects AB produced in D. show that BC = BD.
Tangent at P to the circumcircle of triangle PQR is drawn. If the tangent is parallel to side, QR show that ΔPQR is isosceles.
Circles with centres P and Q intersect at points A and B as shown in the figure. CBD is a line segment and EBM is tangent to the circle, with centre Q, at point B. If the circle are congruent; show that CE = BD.

ABC is a right triangle with angle B = 90°, A circle with BC as diameter meets hypotenuse AC at point D. Prove that: AC × AD = AB2
PT is a tangent to the circle at T. If ∠ ABC = 70° and ∠ ACB = 50° ; calculate : ∠ APT
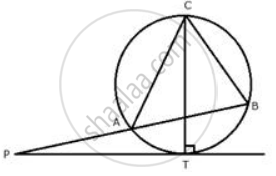
In Fig. AP is a tangent to the circle at P, ABC is secant and PD is the bisector of ∠BPC. Prove that ∠BPD = `1/2` (∠ABP - ∠APB).
In the Figure, PT is a tangent to a circle. If m(∠BTA) = 45° and m(∠PTB) = 70°. Find m(∠ABT).

The figure shows a circle of radius 9 cm with 0 as the centre. The diameter AB produced meets the tangent PQ at P. If PA = 24 cm, find the length of tangent PQ:

