Advertisements
Advertisements
Question
The diameter and a chord of a circle have a common end-point. If the length of the diameter is 20 cm and the length of the chord is 12 cm, how far is the chord from the centre of the circle?
Solution
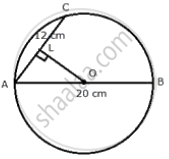
AB is the diameter and AC is the chord.
Draw OL ⊥ AC
Since OL ⊥ AC and hence it bisects AC, O is the centre of the circle.
Therefore, OA = 10 cm and AL = 6 cm
Now, in right ΔOLA,
AO2 = AL2 + OL2
`=>` 102 = 62 + OL2
`=>` OL2 = 100 – 36 = 64
`=>` OL = 8 cm
Therefore, chord is at a distance of 8 cm from the centre of the circle.
APPEARS IN
RELATED QUESTIONS
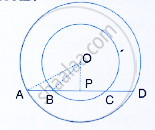
In the following figure, AD is a straight line. OP ⊥ AD and O is the centre of both the circles. If OA = 34 cm. OB = 20 cm and OP = 16cm; find the length of AB.
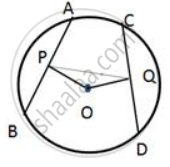
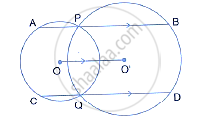
In the following figure; P and Q are the points of intersection of two circles with centres O and O’. If straight lines APB and CQD are parallel to O O'; prove that:
(i) O O' = `1/2AB` (ii) AB = CD
The length of common chord of two intersecting circles is 30 cm. If the diameters of these two circles be 50 cm and 34 cm, calculate the distance between their centres.
In the figure, AB is common chord of the two circles. If AC and AD are diameters; prove that D, B and C are in a straight line. O1 and O2 are the centers of two circles.

In the figure, given alongside, AOB is a diameter of the circle and ∠AOC = 110°. Find ∠BDC.
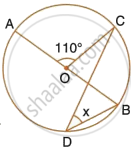
In the figure, AB is the chord of a circle with centre O and DOC is a line segment such that BC = DO. If ∠C = 20°, find angle AOD.

Two circles are drawn with sides AB, AC of a triangle ABC as diameters. They intersect at a point D. Prove that D lies on BC.
In following figure . O is the centre of the circle. Find ∠ BAC.
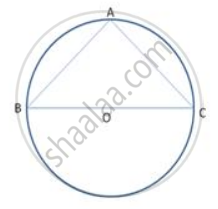
In following figure , chord ED is parallel to the diameter AC of the circle. Given ∠ CBE = 65° , calculate ∠DEC .
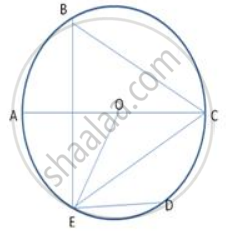
In the given figure, AB and CD are two equal chords of a circle, with centre O. If P is the mid-point of chord AB, Q is the mid-point of chord CD and ∠POQ = 150°, find ∠APQ.