Advertisements
Advertisements
Question
OABC is a rhombus whose three vertices A, B and C lie on a circle with centre O. If the area of the rhombus is `32sqrt(3) cm^2` find the radius of the circle.
Solution

Area of rhombus = `32sqrt(3) cm^2`
But area of rhombus OABC = 2 × area of ΔOAB
Area of rhombus OABC = `2 xx sqrt(3)/4 r^2`
Where r is the side of the equilateral triangle OAB.
`2 xx sqrt(3)/4 r^2 = 32sqrt(3)`
`=> sqrt(3)/2 r^2 = 32sqrt(3)`
`=>` r2 = 64
`=>` r = 8
Therefore, radius of the circle = 8 cm
APPEARS IN
RELATED QUESTIONS
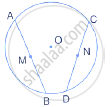
M and N are the mid-points of two equal chords AB and CD respectively of a circle with centre O. prove that:
(i) ∠BMN = ∠DNM.
(ii) ∠AMN = ∠CNM.
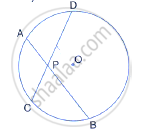
Two equal chords AB and CD of a circle with centre O, intersect each other at point P inside the circle, prove that:
(i) AP = CP,
(ii) BP = DP
OABC is a rhombus whose three vertices A, B and C lie on a circle with centre O. If the radius of the circle is 10 cm, find the area of the rhombus.
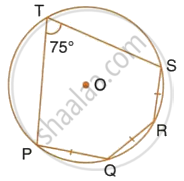
The given figure shows a circle with centre O. Also, PQ = QR = RS and ∠PTS = 75°.
Calculate:
- ∠POS,
- ∠QOR,
- ∠PQR.
In fig, AB and CD are two equal chords of a circle with centre O. If M and N are the midpoints of AB and CD respectively,
prove that (a) ∠ ONM = ∠ ONM (b) ∠ AMN = ∠ CNM.
AB and AC are two equal chords of a circle with centre o such that LABO and LCBO are equal. Prove that AB = BC.
In fig., chords AB and CD of a circle intersect at P. AP = 5cm, BP= 3cm and CP = 2.5cm. Determine the length of DP.
Two equal circles intersect in P and Q. A straight line through P meets the circles in A and B. Prove that QA = QB
In the adjoining diagram, chords AB, BC and CD are equal. O is the centre of the circle. If ∠ ABC = 120°, Calculate: (i) ∠ BAC, (ii) ∠ BEC, (iii) ∠ BED, (iv) ∠ COD
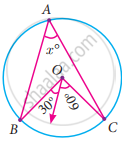
Find the value of x° in the following figure: