Advertisements
Advertisements
Question
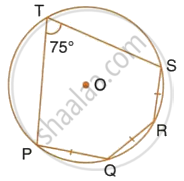
The given figure shows a circle with centre O. Also, PQ = QR = RS and ∠PTS = 75°.
Calculate:
- ∠POS,
- ∠QOR,
- ∠PQR.
Solution
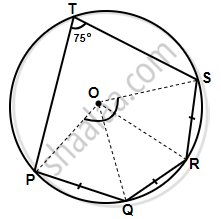
Join OP, OQ and OS.
∵ PQ = QR = RS,
∠POQ = ∠QOR = ∠ROS ...[Equal chords subtends equal angles at the centre]
Arc PQRS subtends ∠POS at the center and ∠PTS at the remaining parts of the circle.
∴ ∠POS = 2∠PTS = 2 × 75° = 150°
`=>` ∠POQ + ∠QOR + ∠ROS = 150°
`=> ∠POQ = ∠QOR = ∠ROS = (150^circ)/3 = 50^circ`
In ΔOPQ, OP = OQ ...[Radii of the same circle]
∴ ∠OPQ = ∠OQP
But ∠OPQ + ∠OQP + ∠POQ = 180°
∴ ∠OPQ + ∠QP = 50° = 180°
`=>` ∠OPQ + ∠OQP = 180° – 50°
`=>` ∠OPQ + ∠OPQ = 130°
`=>` 2∠OPQ = 130°
`=> ∠OPQ = ∠OQP = (130^circ)/2 = 65^circ`
Similarly, we can prove that
In ΔOQR, ∠OQR = ∠ORQ = 65°
And in ΔORS, ∠ORS = OSR = 65°
- Now ∠POS = 150°
- QOR = 50° and
- ∠PQR = ∠PQO + ∠OQR = 65° + 65° = 130°
APPEARS IN
RELATED QUESTIONS
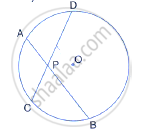
Two equal chords AB and CD of a circle with centre O, intersect each other at point P inside the circle, prove that:
(i) AP = CP,
(ii) BP = DP
OABC is a rhombus whose three vertices A, B and C lie on a circle with centre O. If the radius of the circle is 10 cm, find the area of the rhombus.
In a circle, with centre O, a cyclic quadrilateral ABCD is drawn with AB as a diameter of the circle and CD equal to radius of the circle. If AD and BC produced meet at point P; show that ∠APB = 60°.
In fig. the centre of the circle is O. PQ and RS are two equal chords of the circle which , when produced , meet at T outside the circle . Prove that (a) TP = TR (b) TQ = TS.

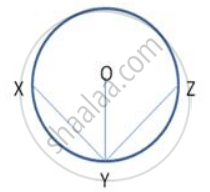
In following figure .,XY and YZ are two equal chords of a circle with centre O. Prove that the bisector of ∠ XYZ passes through O.
In the given Figure, AB and CD are two chords of a circle, intersecting each other at P such that AP = CP. Show that AB= CD.
Two equal chords AB and CD of a circle with center O, intersect each other at point P inside the circle.
Prove that: (i) AP = CP ; (ii) BP = DP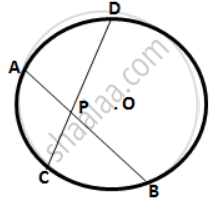
Two equal circles intersect in P and Q. A straight line through P meets the circles in A and B. Prove that QA = QB
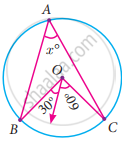
In the adjoining diagram, chords AB, BC and CD are equal. O is the centre of the circle. If ∠ ABC = 120°, Calculate: (i) ∠ BAC, (ii) ∠ BEC, (iii) ∠ BED, (iv) ∠ COD
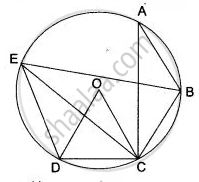
Find the value of x° in the following figure: