Advertisements
Advertisements
Question
Bisectors of vertex angles A, B, and C of a triangle ABC intersect its circumcircle at the points D, E and F respectively. Prove that angle EDF = 90° – `1/2` ∠A.
Solution
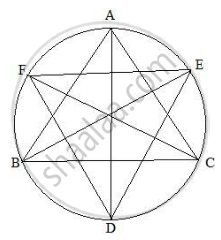
We have,
Given that, BE is the bisector of B.
`therefore angle ABE = (angleB)/2`
`angleADE = angleABE` ...(Angles in the same segment for chord AF)
Similarly, `angle ACF = angleADF = (angleC)/2` ...(Angle in the same segment for chord AF)
`angleD = angleADE + angleADF`
`angleD = (angleB)/2 + (angleC)/2`
= `1/2 (angleB + angleC)`
= `1/2 (180^circ - angleA)` ...`(∴ angleA + angleB + angleC = 180^circ)`
`angleD = 90^circ - (angleA)/2`
APPEARS IN
RELATED QUESTIONS
In the following figure,
- if ∠BAD = 96°, find ∠BCD and ∠BFE.
- Prove that AD is parallel to FE.

In the given figure, AB = AC. Prove that DECB is an isosceles trapezium.
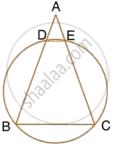
In a cyclic quadrilateral ABCD, the diagonal AC bisects the angle BCD. Prove that the diagonal BD is parallel to the tangent to the circle at point A.
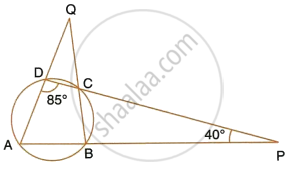
Use the given figure to find:
- ∠BAD,
- ∠DQB.
In the following figure, ABCD is a cyclic quadrilateral in which AD is parallel to BC.

If the bisector of angle A meets BC at point E and the given circle at point F, prove that:
- EF = FC
- BF = DF
In a cyclic quadrilateral ABCD , AB || CD and ∠ B = 65 ° , find the remaining angles

Prove that the angles bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (provided they are not parallel) intersect at right triangle.
If ABCD is a cyclic quadrilateral in which AD || BC. Prove that ∠B = ∠C.
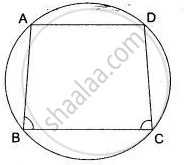
Prove that the angle bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (Provided they are not parallel) intersect at the right angle.
An exterior angle of a cyclic quadrilateral is congruent to the angle opposite to its adjacent interior angle, to prove the theorem complete the activity.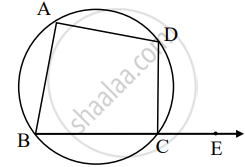
Given: ABCD is cyclic,
`square` is the exterior angle of ABCD
To prove: ∠DCE ≅ ∠BAD
Proof: `square` + ∠BCD = `square` .....[Angles in linear pair] (I)
ABCD is a cyclic.
`square` + ∠BAD = `square` ......[Theorem of cyclic quadrilateral] (II)
By (I) and (II)
∠DCE + ∠BCD = `square` + ∠BAD
∠DCE ≅ ∠BAD
