Advertisements
Advertisements
Question
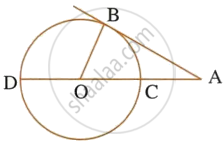
In the given figure, O is the center of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm, calculate the radius of the circle.
Solution 1
AB = 15 cm, AC = 7.5 cm
Let 'r' be the radius of the circle.
∴ OC = OB = r
AO = AC + OC = 7.5 + r
In ΔAOB,
AO2 = AB2 = OB2
(7.5 + r)2 = 152 + r2
`=> ((15 + 2r)/2)^2 = 225 + r^2`
`=>` 225 + 4r2 + 60r = 900 + 4r2
`=>` 60r = 675
`=>` r = 11.25 cm
Therefore, r = 11.25 cm
Solution 2
AB2 = AC × AD ...(PT2 = PA × PB)
`=>` 152 = 7.5 × AD
`=>` AD = `225/7.5` = 30
`=>` CD = AD – AC
= 30 – 7.5
= 22.5
∴ Radius = `1/2` × CD
Radius = `1/2` × 22.5
Radius = 11.25 cm
APPEARS IN
RELATED QUESTIONS
In the following figure; If AB = AC then prove that BQ = CQ.

Tangents AP and AQ are drawn to a circle, with centre O, from an exterior point A. Prove that : ∠PAQ = 2∠OPQ
In a triangle ABC, the incircle (centre O) touches BC, CA and AB at points P, Q and R respectively. Calculate:
i)`∠`QPR .
In the given figure, PT touches the circle with centre O at point R. Diameter SQ is produced to meet the tangent TR at P. Given ∠SPR = x° and ∠QRP = y°;
Prove that:
- ∠ORS = y°
- write an expression connecting x and y.
Tangent at P to the circumcircle of triangle PQR is drawn. If the tangent is parallel to side, QR show that ΔPQR is isosceles.
TA and TB are tangents to a circle with centre O from an external point T. OT intersects the circle at point P. Prove that AP bisects the angle TAB.
In Fig. l and m are two parallel tangents at A and B. The tangent at C makes an intercept DE between n and m. Prove that ∠ DFE = 90°
A, B, and C are three points on a circle. The tangent at C meets BN produced at T. Given that ∠ ATC = 36° and ∠ ACT = 48°, calculate the angle subtended by AB at the center of the circle.
In the joining figure shown XAY is a tangent. If ∠ BDA = 44°, ∠ BXA = 36°.
Calculate: (i) ∠ BAX, (ii) ∠ DAY, (iii) ∠ DAB, (iv) ∠ BCD.

In the adjoining diagram TA and TB are tangents, O is the centre. If ∠ PAT = 35° and ∠ PBT = 40°.
Calculate:
(i) ∠ AQP, (ii) ∠ BQP
(iii) ∠ AQB, (iv) ∠ APB
(v) ∠ AOB, (vi) ∠ ATB