Advertisements
Advertisements
Question
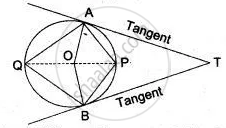
In the adjoining diagram TA and TB are tangents, O is the centre. If ∠ PAT = 35° and ∠ PBT = 40°.
Calculate:
(i) ∠ AQP, (ii) ∠ BQP
(iii) ∠ AQB, (iv) ∠ APB
(v) ∠ AOB, (vi) ∠ ATB
Solution
(i) ∠ AQP = ∠ PAT = 35° ....( Angles are in alternate segment)
(ii) ∠ BQP = ∠ PBT = 40° ....( Angles are in alternate segment)
(iii) ∠ AQB = ∠ AQP + ∠ BQP
∠ AQB = 35° + 40° = 75°
(iv) ∠ APB + ∠ AQB = 180° ....(Opposite ∠s of a cyclic quadrilateral are supplementary)
∴ ∠ APB + 75° = 180°
∴ ∠ APB = 105°
(v) ∠ AOB = 2∠ AQB = 2(75°) = 150° ....(Angle at the centre = 2 Angle at the circumference)
(vi) In quadrilateral AOBT:
∠ ATB = 360° - (∠OAT + ∠OBT + ∠AOB)
∠ ATB = 360° - (90° + 15° + 90°) = 30° ....(∠OAT = ∠OBT = 90° radius, ⊥ tangent).
APPEARS IN
RELATED QUESTIONS
From a point P outside a circle, with centre O, tangents PA and PB are drawn. Prove that:
∠AOP = ∠BOP
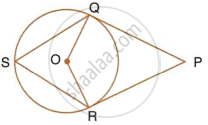
In a triangle ABC, the incircle (centre O) touches BC, CA and AB at points P, Q and R respectively. Calculate:
i)`∠`QPR .
In the following figure, PQ and PR are tangents to the circle, with centre O. If `∠`QPR = 60°, calculate:
- ∠QOR,
- ∠OQR,
- ∠QSR.
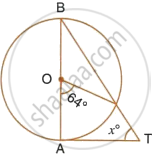
In the given figure, AB is the diameter of the circle, with centre O, and AT is the tangent. Calculate the numerical value of x.
In quadrilateral ABCD; angles D = 90°, BC = 38 cm and DC = 25 cm. A circle is inscribed in this quadrilateral which touches AB at point Q such that QB = 27 cm, Find the radius of the circle.
PT is a tangent to the circle at T. If ∠ABC = 70° and ∠ACB = 50°; calculate:
- ∠CBT
- ∠BAT
- ∠APT

In the given figure, PT touches a circle with centre O at R. Diameter SQ when produced to meet the tangent PT at P. If ∠SPR = x° and ∠QRP = y°; Show that x° + 2y° = 90°

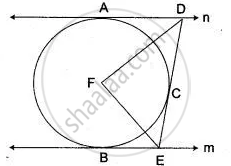
In Fig. l and m are two parallel tangents at A and B. The tangent at C makes an intercept DE between n and m. Prove that ∠ DFE = 90°
In Fig. AT is a tangent to the circle. If m∠ABC = 50°, AC = BC, Find ∠BAT.

In the given figure, AC is a tangent to circle at point B. ∆EFD is an equilateral triangle and ∠CBD = 40°. Find:
- ∠BFD
- ∠FBD
- ∠ABF
