Advertisements
Advertisements
Question
Match the following:
| Column A | Column B |
| (i) No sides are equal | Isosceles triangle |
| (ii) One right angle | Scalene triangle |
| (iii) One obtuse angle | Right angled triangle |
| (iv) Two sides of equal length | Equilateral triangle |
| (v) All sides are equal | Obtuse angled triangle |
Solution
| Column A | Column B |
| (i) No sides are equal | Scalene triangle |
| (ii) One right angle | Right angled triangle |
| (iii) One obtuse angle | Obtuse angled triangle |
| (iv) Two sides of equal length | Isosceles triangle |
| (v) All sides are equal | Equilateral triangle |
APPEARS IN
RELATED QUESTIONS
Is the following statement true and false :
A triangle can have two obtuse angles.
In a Δ ABC, the internal bisectors of ∠B and ∠C meet at P and the external bisectors of ∠B and ∠C meet at Q, Prove that ∠BPC + ∠BQC = 180°.
If the sides of a triangle are produced in order, then the sum of the three exterior angles so formed is
Find x, if the angles of a triangle is:
x°, 2x°, 2x°
Find, giving a reason, the unknown marked angles, in a triangle drawn below:
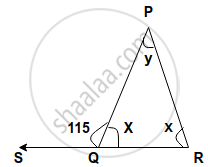
One of the angles of a triangle is 65°. If the difference of the other two angles is 45°, then the two angles are
If an angle of a triangle is equal to the sum of the other two angles, find the type of the triangle
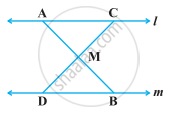
In the following figure, l || m and M is the mid-point of a line segment AB. Show that M is also the mid-point of any line segment CD, having its end points on l and m, respectively.
In figure, AB = BC and AD = BD = DC. The number of isosceles triangles in the figure is ______.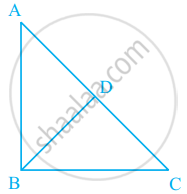
What is common in the following figure?
 |
 |
| (i) | (ii) |
Is figure (i) that of triangle? if not, why?
