Question

If the sides of a triangle are produced in order, then the sum of the three exterior angles so formed is

Options

• 90°

• 180°

• 270°

• 360°

Answer 1

n the given ΔABC, all the three sides of the triangle are produced. We need to find the sum of the three exterior angles so produced.

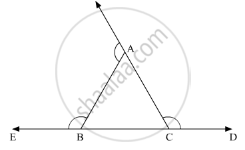
Now, according to the angle sum property of the triangle

∠A + ∠B + ∠C = 180°         .......(1)

Further, using the property, “an exterior angle of the triangle is equal to the sum of two opposite interior angles”, we get,

 ext.∠C = ∠A + ∠B           ......(2)

Similarly, 

 ext.∠A = ∠C + ∠B            .......(3)

Also,    

 ext.∠B = ∠C+ ∠A            .......(4)

Adding (2) (3) and (4)

We get,

 ext.∠A + ext.∠B+ ext.∠C = ∠C+∠B +∠C +∠A + ∠B + ∠A      

 = 2(∠A= ∠B+ ∠C   )

= 2 (180°)

 = 360°

Thus,   ext. ∠A + ext. ∠B ext.∠C  = 360°