Advertisements
Advertisements
Question
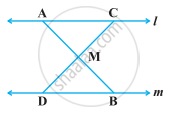
In the following figure, l || m and M is the mid-point of a line segment AB. Show that M is also the mid-point of any line segment CD, having its end points on l and m, respectively.
Solution
Given: In the following figure, l || m and M is the mid-point of a line segment AB i.e., AM = BM.
To show: MC = MD
Proof: l || m ...[Given]
∠BAC = ∠ABD ...[Alternate interior angles]
∠AMC = ∠BMD ...[Vertically opposite angles]
In ΔAMC and ΔBMD,
∠BAC = ∠ABD ...[Proved above]
AM = BM ...[Given]
And ∠AMC = ∠BMD ...[Proved above]
∴ ΔAMC ≅ ΔBMD ...[By ASA congruence rule]
⇒ MC = MD ...[By CPCT]
APPEARS IN
RELATED QUESTIONS
ABC is a triangle in which ∠A — 72°, the internal bisectors of angles B and C meet in O.
Find the magnitude of ∠BOC.
Determine the measure of each of the equal angles of a right-angled isosceles triangle.
OR
ABC is a right-angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
Is the following statement true and false :
An exterior angle of a triangle is equal to the sum of the two interior opposite angles.
Classify the following triangle according to sides:
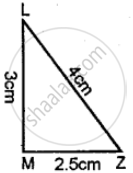
Classify the following triangle according to sides:

The length of the sides of the triangle is given. Say what types of triangles they are 3.4 cm, 3.4 cm, 5 cm.
The length of the sides of the triangle is given. Say what types of triangles they are 4.3 cm, 4.3 cm, 4.3 cm.
One of the angles of a triangle is 65°. If the difference of the other two angles is 45°, then the two angles are
Q is a point on the side SR of a ∆PSR such that PQ = PR. Prove that PS > PQ.
Draw a rough sketch of a triangle ABC. Mark a point P in its interior and a point Q in its exterior. Is point A in its exterior or in its interior?
