Advertisements
Advertisements
Question
Prove by vector method that an angle in a semi-circle is a right angle
Solution
Let AB be the diameter of the circle with centre ‘O’
Let P be any point on the semi-circle.
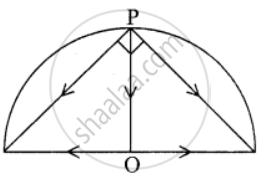
To prove ∠APB = 90°
We have OA = OB = OP .......(radii)
Now `bar"PA" - bar"PO" + bar"OA"`
`bar"PB" = bar"PO" + bar"OB"`
= `bar"PO" - bar"OA"` .....`("Since" bar"OB" = - bar"OA")`
∴ `bar"PA" * bar"PB" = (bar"PO" + bar"OA") * (bar"PO" - bar"OA")`
= `(bar"PO")^2 - (bar"OA")^2`
= (PO)2 – (OA)2
`bar"PA" * bar"PB"` = 0
∴ `bar"PA"` ⊥' to `bar"PB"`
This gives ∠APB = 90°.
Hence the result.
APPEARS IN
RELATED QUESTIONS
Prove by vector method that the median to the base of an isosceles triangle is perpendicular to the base
Prove by vector method that the diagonals of a rhombus bisect each other at right angles
Using vector method, prove that if the diagonals of a parallelogram are equal, then it is a rectangle
Prove by vector method that the parallelograms on the same base and between the same parallels are equal in area
Using vector method, prove that cos(α – β) = cos α cos β + sin α sin β
Forces of magnitudes `5sqrt(2)` and `10sqrt(2)` units acting in the directions `3hat"i" + 4hat"j" + 5hat"k"` and `10hat"i" + 6hat"j" - 8hat"k"` respectively, act on a particle which is displaced from the point with position vector `4hat"i" - 3hat"j" - 2hat"k"` to the point with position vector `6hat"i" + hat"j" - 3hat"k"`. Find the work done by the forces
Find the torque of the resultant of the three forces represented by `- 3hat"i" + 6hat"j" - 3hat"k", 4hat"i" - 10hat"j" + 12hat"k"` and `4hat"i" + 7hat"j"` acting at the point with position vector `8hat"i" - 6hat"j" - 4hat"k"` about the point with position vector `18hat"i" + 3hat"j" - 9hat"k"`
Choose the correct alternative:
If `vec"a"` and `vec"b"` are parallel vectors, then `[vec"a", vec"c", vec"b"]` is equal to
Let `veca = αhati + 3hatj - hatk, vecb = 3hati - βhatj + 4hatk` and `vecc = hati + 2hatj - 2hatk` where α, β ∈ R, be three vectors. If the projection of a `veca` on `vecc` is `10/3` and `vecb xx vecc = -6hati + 10hatj + 7hatk`, then the value of α + β is equal to ______.
Let `veca = hati - 2hatj + 3hatk, vecb = hati + hatj + hatk` and `vecc` be a vector such that `veca + (vecb xx vecc) = vec0` and `vecb.vecc` = 5. Then, the value of `3(vecc.veca)` is equal to ______.
Let `veca = 2hati + hatj - 2hatk` and `vecb = hati + hatj`. If `vecc` is a vector such that `veca.vecc = |vecc|, |vecc - veca| = 2sqrt(2)`, angle between `(veca xx vecb)` and `vecc` is `π/6`, then the value of `|(veca xx vecb) xx vecc|` is ______.
Let `veca, vecb, vecc` be three mutually perpendicular vectors of the same magnitude and equally inclined at an angle θ, with the vector `veca + vecb + vecc`. Then, 36 cos22θ is equal to ______.
Let `veca, vecb` and `vecc` be three unit vectors such that `|veca - vecb|^2 + |veca - vecc|^2` = 8. Then find the value of `|veca + 2vecb|^2 + |veca + 2vecc|^2`
The value of `[veca + 2vecb - vecc, veca - vecb, veca - vecb - vecc]` is equal to the box product ______.
Let a, b, c ∈ R be such that a2 + b2 + c2 = 1. If `a cos θ = b cos(θ + (2π)/3) = c cos(θ + (4π)/3)`, where θ = `π/9`, then the angle between the vectors `ahati + bhatj + chatk` and `bhati + chatj + ahatk` is ______.
If `vecx` and `vecy` be two non-zero vectors such that `|vecx + vecy| = |vecx|` and `2vecx + λvecy` is perpendicular to `vecy`, then the value of λ is ______.
