Advertisements
Advertisements
Question
Represent to solution set of each of the following inequations graphically in two dimensional plane:
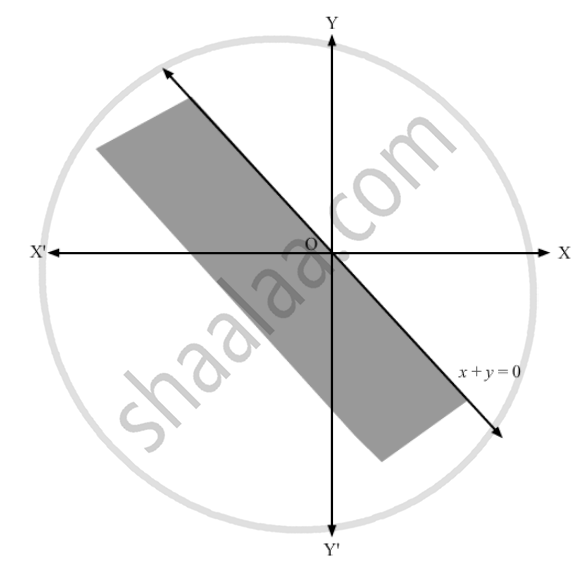
x + 2y − y ≤ 0
Solution
\[\text{ We have }, \]
\[x + 2y - y \leq 0\]
Converting the given inequation to equation, we obtain x + 2y\[-\] y = 0, i.e x + y = 0
So, this line intersects the x-axis and the y-axis at (0,0).
We draw the line of the equation x + y = 0
Now we take a point (1, 1) ( any point which does not lie on the line x + y = 0 )
(1, 1) does not satisfy the inequality. So, the region not containing (1, 1)
is represented by the following figure.
Hence, the shaded region represents the in equation.
APPEARS IN
RELATED QUESTIONS
Solve 24x < 100, when x is a natural number.
Solve 24x < 100, when x is an integer.
Solve –12x > 30, when x is an integer.
Solve 5x – 3 < 7, when x is a real number.
Solve 3x + 8 > 2, when x is a real number.
Solve the given inequality for real x: 3x – 7 > 5x – 1.
Solve the given inequality for real x: 3(x – 1) ≤ 2 (x – 3)
Solve the given inequality for real x: 3(2 – x) ≥ 2(1 – x)
Solve the given inequality for real x: x + `x/2` + `x/3` < 11
Solve the given inequality for real x : `x/3 > x/2 + 1`
Solve the given inequality for real x: `1/2 ((3x)/5 + 4) >= 1/3 (x -6)`
Solve the given inequality for real x: 2(2x + 3) – 10 < 6 (x – 2)
Solve the given inequality for real x: 37 – (3x + 5) ≥ 9x – 8(x – 3)
Solve the given inequality and show the graph of the solution on number line:
3(1 – x) < 2 (x + 4)
Solve the given inequality and show the graph of the solution on number line:
`x/2 >= ((5x -2))/3 - ((7x - 3))/5`
Find all pairs of consecutive odd positive integers both of which are smaller than 10 such that their sum is more than 11.
The longest side of a triangle is 3 times the shortest side and the third side is 2 cm shorter than the longest side. If the perimeter of the triangle is at least 61 cm, find the minimum length of the shortest side.
Solve the inequality.
6 ≤ –3(2x – 4) < 12
Solve the inequality.
`-15 < (3(x - 2))/5 <= 0`
Solve the inequality.
`-12 < 4 - (3x)/(-5) <= 2`
Solve the inequality.
`7 <= (3x + 11)/2 <= 11`
Represent to solution set of each of the following inequations graphically in two dimensional plane:
x + 2 ≥ 0
Represent to solution set of each of the following inequations graphically in two dimensional plane:
4. x − 2y < 0
Represent to solution set of each of the following inequations graphically in two dimensional plane:
3y ≥ 6 − 2x
State whether the following statement is True or False.
If x > y and b < 0, then bx < by
State whether the following statement is True or False.
If xy > 0, then x > 0, and y < 0
State whether the following statement is True or False.
If xy < 0, then x > 0, and y > 0
State whether the following statement is True or False.
If x > 5 and x > 2, then x ∈ (5, ∞)
State whether the following statement is True or False.
If |x| < 5, then x ∈ (–5, 5)
The inequality representing the following graph is ______.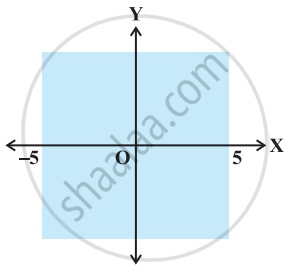
Solution of a linear inequality in variable x is represented on number line given below ______.

If x > –2 and x < 9, then x ∈ (– 2, 9)
If |x| > 5, then x ∈ (– `oo`, – 5) ∪ [5, `oo`)
