Advertisements
Advertisements
Question
Show that points A(–1, –1), B(0, 1), C(1, 3) are collinear.
Solution 1
A(–1, –1), B(0, 1), C(1, 3)
AB = `sqrt((0 + 1)^2 + (1 + 1)^2`
= `sqrt(1 + 4)`
AB = `sqrt(5)`
BC = `sqrt((1)^2 + (2)^2`
= `sqrt(1 + 4)`
BC = `sqrt(5)`
AC = `sqrt((2)^2 + (3 + 1)^2`
= `sqrt(4 + 16)`
= `sqrt(20)`
= `sqrt(5 xx 4)`
AC = `2sqrt(5)`
AB + BC = AC
`sqrt(5) + sqrt(5) = 2sqrt(5)`
A, B, and C are collinear.
Solution 2
A`(x_1, y_1) = (-1, -1)`
B`(x_2, y_2) = (0,1)`
C`(x_3, y_3) = (1, 3)`
Slope of line
AB = `(y_2 - y_1)/(x_2 - x_1)`
= `(1 - (-1))/(0 - (-1))`
= `(1+1)/1 = 2`
Slope of line
BC = `(y_3 - y_2)/(x_3 - x_2)`
= `(3 - 1)/(1 - 0)`
= `2/1 = 2.`
As, slope of line AB = slope of line BC
Also AB and BC Hrtes contain common point B
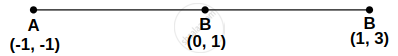
∴ Points A, B, C are collinear. Hence Proved
APPEARS IN
RELATED QUESTIONS
If two vertices of an equilateral triangle be (0, 0), (3, √3 ), find the third vertex
Find the distance between the following pairs of points:
(−5, 7), (−1, 3)
Check whether (5, -2), (6, 4) and (7, -2) are the vertices of an isosceles triangle.
Find the point on the x-axis which is equidistant from (2, -5) and (-2, 9).
Find the distance between the following pair of points:
(asinα, −bcosα) and (−acos α, bsin α)
An equilateral triangle has two vertices at the points (3, 4) and (−2, 3), find the coordinates of the third vertex.
Find all possible values of y for which distance between the points is 10 units.
If the point A(x,2) is equidistant form the points B(8,-2) and C(2,-2) , find the value of x. Also, find the value of x . Also, find the length of AB.
Find the distance between the following pair of point.
P(–5, 7), Q(–1, 3)
Show that the ▢PQRS formed by P(2, 1), Q(–1, 3), R(–5, –3) and S(–2, –5) is a rectangle.
If the point P(2, 1) lies on the line segment joining points A(4, 2) and B(8, 4), then ______.
Find the distance of the following point from the origin :
(5 , 12)
Find the point on the x-axis equidistant from the points (5,4) and (-2,3).
Show that the points P (0, 5), Q (5, 10) and R (6, 3) are the vertices of an isosceles triangle.
Find the distance of the following points from origin.
(a cos θ, a sin θ).
Calculate the distance between A (7, 3) and B on the x-axis, whose abscissa is 11.
Find distance between points O(0, 0) and B(– 5, 12)
Show that the points (2, 0), (– 2, 0) and (0, 2) are vertices of a triangle. State the type of triangle with reason
AOBC is a rectangle whose three vertices are A(0, 3), O(0, 0) and B(5, 0). The length of its diagonal is ______.
