Question

Solve the following problem.

A 2 m long ladder of mass 10 kg is kept against a wall such that its base is 1.2 m away from the wall. The wall is smooth but the ground is rough. The roughness of the ground is such that it offers a maximum horizontal resistive force (for sliding motion) half that of normal reaction at the point of contact. A monkey of mass 20 kg starts climbing the ladder. How far can it climb along the ladder? How much is the horizontal reaction at the wall?

Answer 1

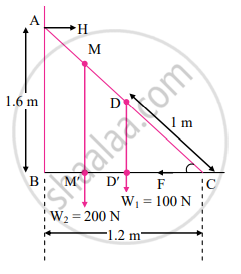
From the figure,

Given that, AC = length of ladder = 2 m

BC = 1.2 m

From Pythagoras theorem,

AB = `sqrt("AC"^2 - "BC"^2) = 1.6`m    .....(i)

Also, ΔABC ~ Δ DD'C

∴ `"AB"/"DD'" = "BC"/"D'C" = "AC"/"DC"`

∴ `1.2/"D'C" = 2/1`

∴ D'C = 0.6 m             ....(ii)

The ladder exerts horizontal force `vec"H"` on the wall at A and `vec"F"` is the force exerted on the ground at C.

As `|vec"F"| = "N"/2, |vec"H"| = |vec"F"| = "N"/2`    ....(iii)

Let monkey climb upto distance x along BC (Horizontal) i.e., CM' = x ….(iv)

Then, the net normal reaction at point C will be,
N = 100 + 200 = 300 N

From equation (iii),

H = `"N"/2 = 300/2 = 150 "N"`

By condition of equilibrium, taking moments about C,

(–H × AB) + (W₁ × CD') + (W₂ × CM') + (F × 0) = 0

∴ (- 150 × 1.6) + (100 × 0.6) + (200 × `x`) = 0

∴ 60 + 200x = 240

∴ 200x = 180

∴ x = 0.9

From figure, it can be shown that,

ΔABC ~ ΔMM'C

∴ `"BC"/"CM'" = "AC"/"CM"`

∴ `1.2/0.9 = 2/"CM"`

∴ CM = 1.5 m

i. The monkey can climb upto 1.5 m along the ladder.

ii. The horizontal reaction at wall is 150 N.