Advertisements
Advertisements
Question
Solve the following problem :
Maximize Z = 4x1 + 3x2 Subject to 3x1 + x2 ≤ 15, 3x1 + 4x2 ≤ 24, x1 ≥ 0, x2 ≥ 0
Solution
To find the graphical solution, construct the table as follows:
| Inequation | Equation | Double intercept form | Points (x1, x2) | Region |
| 3x1 + x2 ≤ 15 | 3x1 + x2 = 15 | `x_1/(5) + x_2/(15)` = 1 | A (5, 0) B (0, 15) |
3(0) + 0 ≤ 15 ∴ 0 ≤ 15 ∴ origin side |
| 3x1 + 4x2 ≤ 24 | 3x1 + 4x2 = 24 | `x_1/8 + x_2/(6)` = 1 | C (8, 0) D (0, 6) |
3(0 + 4(0) ≤ 24 ∴ 0 ≤ 24 ∴ origin side |
| x1 ≥ 0 | x1 = 0 | – | – | R.H.S. of Y-axis |
| x2 ≥ 0 | x2 = 0 | – | – | above X-axis |
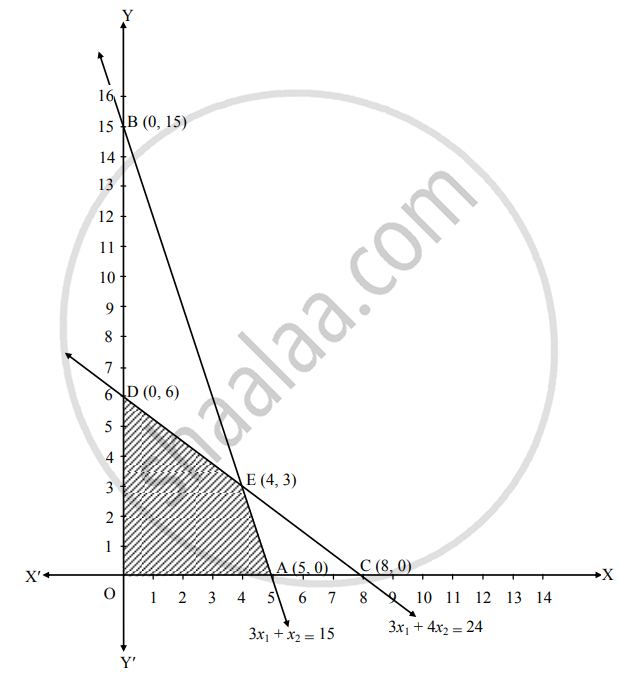

Shaded portion ODEA is the feasible region.
Whose vertices are O (0, 0), D (0, 6), E, A (5, 0)
E is the point of intersection of the lines
3x1 + x2 = 15 …(i)
and 3x1 + 4x2 = 24 …(ii)
∴ By (i) – (ii), we get
3x1 + x2 = 15
3x1 + 4x2 = 24
– – –
–3x2 = – 9
∴ x2 = `(-9)/(-3)`
∴ x2 = 3
Substituting x2 = 3 in i, we get
3x1 + 3 = 15
∴ 3x1 = 15 – 3
∴ 3x1 = 12
∴ x1 = `(12)/(3)` = 4
∴ E (4, 3)
Here, the objective function is Z = 4x1 + 3x2
Now, we will find maximum value of Z as follows:
| Feasible points | The value of Z = 4x1 + 3x2 |
| O (0, 0) | Z = 4(0) + 3(0) = 0 |
| D (0, 6) | Z = 4(0) + 3(0) = 0 |
| E (4, 3) | Z = 4(4) + 3(3) = 16 + 9 = 25 |
| A (5, 0) | Z = 4(4) + 3(3) = 16 + 9 = 25 |
∴ Z has maximum value 25 at E(4, 3)
∴ Z is maximum, when x1 = 4, x2 = 3.
APPEARS IN
RELATED QUESTIONS
A firm manufactures 3 products A, B and C. The profits are Rs 3, Rs 2 and Rs 4 respectively. The firm has 2 machines and below is the required processing time in minutes for each machine on each product :
| Machine | Products | ||
| A | B | C | |
| M1 M2 |
4 | 3 | 5 |
| 2 | 2 | 4 | |
Machines M1 and M2 have 2000 and 2500 machine minutes respectively. The firm must manufacture 100 A's, 200 B's and 50 C's but not more than 150 A's. Set up a LPP to maximize the profit.
Solve the following L.P.P. by graphical method :
Maximize : Z = 7x + 11y subject to 3x + 5y ≤ 26, 5x + 3y ≤ 30, x ≥ 0, y ≥ 0.
Choose the correct alternative:
The value of objective function is maximize under linear constraints.
Choose the correct alternative :
The maximum value of z = 5x + 3y. subject to the constraints
The region represented by the inequality y ≤ 0 lies in _______ quadrants.
State whether the following is True or False :
The region represented by the inqualities x ≤ 0, y ≤ 0 lies in first quadrant.
A carpenter makes chairs and tables, profits are ₹ 140 per chair and ₹ 210 per table. Both products are processed on three machines, Assembling, Finishing and Polishing. The time required for each product in hours and the availability of each machine is given by the following table.
| Product/Machines | Chair (x) |
Table (y) |
Available time (hours) |
| Assembling | 3 | 3 | 36 |
| Finishing | 5 | 2 | 50 |
| Polishing | 2 | 6 | 60 |
Formulate and solve the following Linear programming problems using graphical method.
Choose the correct alternative:
If LPP has optimal solution at two point, then
Choose the correct alternative:
The point at which the minimum value of Z = 8x + 12y subject to the constraints 2x + y ≥ 8, x + 2y ≥ 10, x ≥ 0, y ≥ 0 is obtained at the point
Choose the correct alternative:
The corner points of feasible region for the inequations, x + y ≤ 5, x + 2y ≤ 6, x ≥ 0, y ≥ 0 are
State whether the following statement is True or False:
Of all the points of feasible region, the optimal value is obtained at the boundary of the feasible region
State whether the following statement is True or False:
The graphical solution set of the inequations 0 ≤ y, x ≥ 0 lies in second quadrant
A dealer deals in two products X and Y. He has ₹ 1,00,000/- to invest and space to store 80 pieces. Product X costs ₹ 2500/- and product Y costs ₹ 1000/- per unit. He can sell the items X and Y at respective profits of ₹ 300 and ₹ 90. Construct the LPP and find the number of units of each product to be purchased to maximize its profit
Smita is a diet conscious house wife, wishes to ensure certain minimum intake of vitamins A, B and C for the family. The minimum daily needs of vitamins A, B, and C for the family are 30, 20, and 16 units respectively. For the supply of the minimum vitamin requirements Smita relies on 2 types of foods F1 and F2. F1 provides 7, 5 and 2 units of A, B, C vitamins per 10 grams and F2 provides 2, 4 and 8 units of A, B and C vitamins per 10 grams. F1 costs ₹ 3 and F2 costs ₹ 2 per 10 grams. How many grams of each F1 and F2 should buy every day to keep her food bill minimum
A wholesale dealer deals in two kinds of mixtures A and B of nuts. Each kg of mixture A contains 60 grams of almonds, 30 grams of cashew and 30 grams of hazel nuts. Each kg of mixture B contains 30 grams of almonds, 60 grams of cashew and 180 grams of hazel nuts. A dealer is contemplating to use mixtures A and B to make a bag which will contain at least 240 grams of almonds, 300 grams of cashew and 540 grams of hazel nuts. Mixture A costs ₹ 8 and B costs ₹ 12 per kg. How many kgs of each mixture should he use to minimize the cost of the kgs
Maximize Z = 2x + 3y subject to constraints
x + 4y ≤ 8, 3x + 2y ≤ 14, x ≥ 0, y ≥ 0.
Minimize Z = x + 4y subject to constraints
x + 3y ≥ 3, 2x + y ≥ 2, x ≥ 0, y ≥ 0
A linear function z = ax + by, where a and b are constants, which has to be maximised or minimised according to a set of given condition is called a:-
