Advertisements
Advertisements
Question
A dealer deals in two products X and Y. He has ₹ 1,00,000/- to invest and space to store 80 pieces. Product X costs ₹ 2500/- and product Y costs ₹ 1000/- per unit. He can sell the items X and Y at respective profits of ₹ 300 and ₹ 90. Construct the LPP and find the number of units of each product to be purchased to maximize its profit
Solution
Let the dealer purchase x units of X and y units of Y.
The dealer can sell items X and Y at respective profits of ₹ 300 and ₹ 90
∴ Total profit = Z = 300x + 90y
∴ Given problem can be formulated as follows:
Maximize Z = 300x + 90y
Subject to x + y ≤ 80
2500x + 1000y ≤ 100000, x ≥ 0, y ≥ 0
To draw the feasible region, construct table as follows:
| Inequality | x + y ≤ 80 | 2500x + 1000y ≤ 100000 |
| Corresponding equation (of line) | x + y = 80 | 2500x + 1000y = 100000 |
| Intersection of line with X-axis | (80, 0) | (40, 0) |
| Intersection of line with Y-axis | (0, 80) | (0, 100) |
| Region | Origin side | Origin side |
Shaded portion OABC is the feasible region, whose vertices are O(0, 0), A(40, 0), B and C(0, 80).
B is the point of intersection of the lines 2500x + 1000y = 100000 and x + y = 80.
Solving the above equations, we get
x = `40/3`, y = `200/3`
∴ B ≡ `(40/3, 200/3)`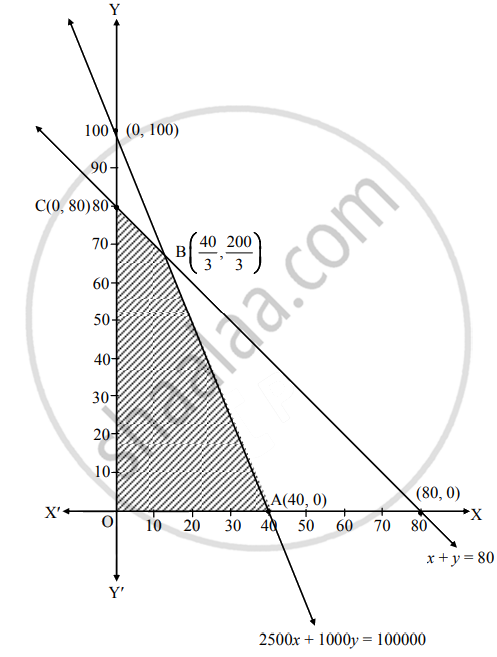
Here, the objective function is
Z = 300x + 90y
∴ Z at O(0, 0) = 300(0) + 90(0) = 0
Z at A(40, 0) = 300 (40) + 90(0)
= 12000
Z at B`(40/3, 200/3) = 300(40/3) + 90(200/3)`
= 4000 + 6000
= 10000
Z at C(0, 80) = 300 (0) + 90(80)
= 7200
∴ Z has maximum value 12000 at x = 40 and y = 0.
∴ The dealer should purchase 40 units of X and 0 units of Y to maximize its profit.
APPEARS IN
RELATED QUESTIONS
A small manufacturing firm produces two types of gadgets A and B, which are first processed in the foundry, then sent to the machine shop for finishing. The number of man-hours of labour required in each shop for the production of each unit of A and B, and the number of man-hours the firm has available per week are as follows:
| Gadget | Foundry | Machine-shop |
| A | 10 | 5 |
| B | 6 | 4 |
| Firm's capacity per week | 1000 | 600 |
The profit on the sale of A is Rs 30 per unit as compared with Rs 20 per unit of B. The problem is to determine the weekly production of gadgets A and B, so that the total profit is maximized. Formulate this problem as a LPP.
A farmer has a 100 acre farm. He can sell the tomatoes, lettuce, or radishes he can raise. The price he can obtain is Rs 1 per kilogram for tomatoes, Rs 0.75 a head for lettuce and Rs 2 per kilogram for radishes. The average yield per acre is 2000 kgs for radishes, 3000 heads of lettuce and 1000 kilograms of radishes. Fertilizer is available at Rs 0.50 per kg and the amount required per acre is 100 kgs each for tomatoes and lettuce and 50 kilograms for radishes. Labour required for sowing, cultivating and harvesting per acre is 5 man-days for tomatoes and radishes and 6 man-days for lettuce. A total of 400 man-days of labour are available at Rs 20 per man-day. Formulate this problem as a LPP to maximize the farmer's total profit.
The corner points of the feasible region determined by the following system of linear inequalities:
2x + y ≤ 10, x + 3y ≤ 15, x, y ≥ 0 are (0, 0), (5, 0), (3, 4) and (0, 5). Let Z = px + qy, where p, q > 0. Condition on p and q so that the maximum of Z occurs at both (3, 4) and (0, 5) is
A firm manufactures two products, each of which must be processed through two departments, 1 and 2. The hourly requirements per unit for each product in each department, the weekly capacities in each department, selling price per unit, labour cost per unit, and raw material cost per unit are summarized as follows:
| Product A | Product B | Weekly capacity | |
| Department 1 | 3 | 2 | 130 |
| Department 2 | 4 | 6 | 260 |
| Selling price per unit | ₹ 25 | ₹ 30 | |
| Labour cost per unit | ₹ 16 | ₹ 20 | |
| Raw material cost per unit | ₹ 4 | ₹ 4 |
The problem is to determine the number of units to produce each product so as to maximize total contribution to profit. Formulate this as a LPP.
Solve the following L.P.P. by graphical method :
Maximize : Z = 7x + 11y subject to 3x + 5y ≤ 26, 5x + 3y ≤ 30, x ≥ 0, y ≥ 0.
Solve the following L.P.P. by graphical method:
Maximize: Z = 10x + 25y
subject to 0 ≤ x ≤ 3,
0 ≤ y ≤ 3,
x + y ≤ 5.
Also find the maximum value of z.
Fill in the blank :
The region represented by the in equations x ≤ 0, y ≤ 0 lines in _______ quadrants.
The region represented by the inequality y ≤ 0 lies in _______ quadrants.
Graphical solution set of x ≤ 0, y ≥ 0 in xy system lies in second quadrant.
A carpenter makes chairs and tables, profits are ₹ 140 per chair and ₹ 210 per table. Both products are processed on three machines, Assembling, Finishing and Polishing. The time required for each product in hours and the availability of each machine is given by the following table.
| Product/Machines | Chair (x) |
Table (y) |
Available time (hours) |
| Assembling | 3 | 3 | 36 |
| Finishing | 5 | 2 | 50 |
| Polishing | 2 | 6 | 60 |
Formulate and solve the following Linear programming problems using graphical method.
Solve the following problem :
A firm manufacturing two types of electrical items A and B, can make a profit of ₹ 20 per unit of A and ₹ 30 per unit of B. Both A and B make use of two essential components, a motor and a transformer. Each unit of A requires 3 motors and 2 transformers and each unit of B requires 2 motors and 4 transformers. The total supply of components per month is restricted to 210 motors and 300 transformers. How many units of A and B should be manufacture per month to maximize profit? How much is the maximum profit?
State whether the following statement is True or False:
If the corner points of the feasible region are (0, 10), (2, 2) and (4, 0), then the minimum value of Z = 3x + 2y is at (4, 0)
State whether the following statement is True or False:
Of all the points of feasible region, the optimal value is obtained at the boundary of the feasible region
If the feasible region is bounded by the inequations 2x + 3y ≤ 12, 2x + y ≤ 8, 0 ≤ x, 0 ≤ y, then point (5, 4) is a ______ of the feasible region
A company manufactures two types of ladies dresses C and D. The raw material and labour available per day is given in the table.
| Resources | Dress C(x) | Dress D(y) | Max. availability |
| Raw material | 5 | 4 | 60 |
| Labour | 5 | 3 | 50 |
P is the profit, if P = 50x + 100y, solve this LPP to find x and y to get the maximum profit
Smita is a diet conscious house wife, wishes to ensure certain minimum intake of vitamins A, B and C for the family. The minimum daily needs of vitamins A, B, and C for the family are 30, 20, and 16 units respectively. For the supply of the minimum vitamin requirements Smita relies on 2 types of foods F1 and F2. F1 provides 7, 5 and 2 units of A, B, C vitamins per 10 grams and F2 provides 2, 4 and 8 units of A, B and C vitamins per 10 grams. F1 costs ₹ 3 and F2 costs ₹ 2 per 10 grams. How many grams of each F1 and F2 should buy every day to keep her food bill minimum
Maximize Z = 2x + 3y subject to constraints
x + 4y ≤ 8, 3x + 2y ≤ 14, x ≥ 0, y ≥ 0.
Minimize Z = x + 4y subject to constraints
x + 3y ≥ 3, 2x + y ≥ 2, x ≥ 0, y ≥ 0
A linear function z = ax + by, where a and b are constants, which has to be maximised or minimised according to a set of given condition is called a:-
Shraddho wants to invest at most ₹ 25,000/- in saving certificates and fixed deposits. She wants to invest at least ₹ 10,000/- in saving certificate and at least ₹ 15,000/- in fixed deposits. The rate of interest on saving certificate is 5% and that on fixed deposits is 7% per annum. Formulate the above problem as LPP to determine maximum income yearly.
