Advertisements
Advertisements
Question
The corner points of the feasible region determined by the following system of linear inequalities:
2x + y ≤ 10, x + 3y ≤ 15, x, y ≥ 0 are (0, 0), (5, 0), (3, 4) and (0, 5). Let Z = px + qy, where p, q > 0. Condition on p and q so that the maximum of Z occurs at both (3, 4) and (0, 5) is
Options
p = q
p = 2q
p = 3q
q = 3p
Solution
q = 3p
It is given that the maximum value of Z occurs at two points (3, 4) and (0, 5).
∴ Value of Z at (3, 4) = Value of Z at (0, 5)
⇒ p(3) + q(4) = p(0) + q(5)
⇒ 3p + 4q = 5q
⇒ q = 3p
APPEARS IN
RELATED QUESTIONS
The postmaster of a local post office wishes to hire extra helpers during the Deepawali season, because of a large increase in the volume of mail handling and delivery. Because of the limited office space and the budgetary conditions, the number of temporary helpers must not exceed 10. According to past experience, a man can handle 300 letters and 80 packages per day, on the average, and a woman can handle 400 letters and 50 packets per day. The postmaster believes that the daily volume of extra mail and packages will be no less than 3400 and 680 respectively. A man receives Rs 225 a day and a woman receives Rs 200 a day. How many men and women helpers should be hired to keep the pay-roll at a minimum ? Formulate an LPP and solve it graphically.
A farmer has a 100 acre farm. He can sell the tomatoes, lettuce, or radishes he can raise. The price he can obtain is Rs 1 per kilogram for tomatoes, Rs 0.75 a head for lettuce and Rs 2 per kilogram for radishes. The average yield per acre is 2000 kgs for radishes, 3000 heads of lettuce and 1000 kilograms of radishes. Fertilizer is available at Rs 0.50 per kg and the amount required per acre is 100 kgs each for tomatoes and lettuce and 50 kilograms for radishes. Labour required for sowing, cultivating and harvesting per acre is 5 man-days for tomatoes and radishes and 6 man-days for lettuce. A total of 400 man-days of labour are available at Rs 20 per man-day. Formulate this problem as a LPP to maximize the farmer's total profit.
A firm manufactures two products, each of which must be processed through two departments, 1 and 2. The hourly requirements per unit for each product in each department, the weekly capacities in each department, selling price per unit, labour cost per unit, and raw material cost per unit are summarized as follows:
| Product A | Product B | Weekly capacity | |
| Department 1 | 3 | 2 | 130 |
| Department 2 | 4 | 6 | 260 |
| Selling price per unit | ₹ 25 | ₹ 30 | |
| Labour cost per unit | ₹ 16 | ₹ 20 | |
| Raw material cost per unit | ₹ 4 | ₹ 4 |
The problem is to determine the number of units to produce each product so as to maximize total contribution to profit. Formulate this as a LPP.
Solve the following LPP by graphical method:
Maximize z = 11x + 8y, subject to x ≤ 4, y ≤ 6, x + y ≤ 6, x ≥ 0, y ≥ 0
Choose the correct alternative:
The value of objective function is maximize under linear constraints.
Choose the correct alternative :
The maximum value of z = 5x + 3y. subject to the constraints
Choose the correct alternative :
The maximum value of z = 10x + 6y, subjected to the constraints 3x + y ≤ 12, 2x + 5y ≤ 34, x ≥ 0, y ≥ 0 is.
The region represented by the inequalities x ≥ 0, y ≥ 0 lies in first quadrant.
State whether the following is True or False :
The region represented by the inqualities x ≤ 0, y ≤ 0 lies in first quadrant.
Solve the following problem :
Minimize Z = 2x + 3y Subject to x – y ≤ 1, x + y ≥ 3, x ≥ 0, y ≥ 0
Solve the following problem :
Maximize Z = 4x1 + 3x2 Subject to 3x1 + x2 ≤ 15, 3x1 + 4x2 ≤ 24, x1 ≥ 0, x2 ≥ 0
Solve the following problem :
Maximize Z = 60x + 50y Subject to x + 2y ≤ 40, 3x + 2y ≤ 60, x ≥ 0, y ≥ 0
Solve the following problem :
A firm manufacturing two types of electrical items A and B, can make a profit of ₹ 20 per unit of A and ₹ 30 per unit of B. Both A and B make use of two essential components, a motor and a transformer. Each unit of A requires 3 motors and 2 transformers and each unit of B requires 2 motors and 4 transformers. The total supply of components per month is restricted to 210 motors and 300 transformers. How many units of A and B should be manufacture per month to maximize profit? How much is the maximum profit?
Choose the correct alternative:
The minimum value of Z = 4x + 5y subjected to the constraints x + y ≥ 6, 5x + y ≥ 10, x, y ≥ 0 is
Choose the correct alternative:
The corner points of the feasible region are (0, 3), (3, 0), (8, 0), `(12/5, 38/5)` and (0, 10), then the point of maximum Z = 6x + 4y = 48 is at
State whether the following statement is True or False:
The maximum value of Z = 5x + 3y subjected to constraints 3x + y ≤ 12, 2x + 3y ≤ 18, 0 ≤ x, y is 20
State whether the following statement is True or False:
If LPP has two optimal solutions, then the LPP has infinitely many solutions
State whether the following statement is True or False:
A convex set includes the points but not the segment joining the points
State whether the following statement is True or False:
If the corner points of the feasible region are `(0, 7/3)`, (2, 1), (3, 0) and (0, 0), then the maximum value of Z = 4x + 5y is 12
State whether the following statement is True or False:
If the corner points of the feasible region are (0, 10), (2, 2) and (4, 0), then the minimum value of Z = 3x + 2y is at (4, 0)
State whether the following statement is True or False:
Of all the points of feasible region, the optimal value is obtained at the boundary of the feasible region
The feasible region represented by the inequations x ≥ 0, y ≤ 0 lies in ______ quadrant.
A dealer deals in two products X and Y. He has ₹ 1,00,000/- to invest and space to store 80 pieces. Product X costs ₹ 2500/- and product Y costs ₹ 1000/- per unit. He can sell the items X and Y at respective profits of ₹ 300 and ₹ 90. Construct the LPP and find the number of units of each product to be purchased to maximize its profit
A company manufactures two types of ladies dresses C and D. The raw material and labour available per day is given in the table.
| Resources | Dress C(x) | Dress D(y) | Max. availability |
| Raw material | 5 | 4 | 60 |
| Labour | 5 | 3 | 50 |
P is the profit, if P = 50x + 100y, solve this LPP to find x and y to get the maximum profit
A chemist has a compound to be made using 3 basic elements X, Y, Z so that it has at least 10 litres of X, 12 litres of Y and 20 litres of Z. He makes this compound by mixing two compounds (I) and (II). Each unit compound (I) had 4 litres of X, 3 litres of Y. Each unit compound (II) had 1 litre of X, 2 litres of Y and 4 litres of Z. The unit costs of compounds (I) and (II) are ₹ 400 and ₹ 600 respectively. Find the number of units of each compound to be produced so as to minimize the cost
Maximize Z = 2x + 3y subject to constraints
x + 4y ≤ 8, 3x + 2y ≤ 14, x ≥ 0, y ≥ 0.
Maximize Z = 400x + 500y subject to constraints
x + 2y ≤ 80, 2x + y ≤ 90, x ≥ 0, y ≥ 0
Minimize Z = x + 4y subject to constraints
x + 3y ≥ 3, 2x + y ≥ 2, x ≥ 0, y ≥ 0
Minimize Z = 2x + 3y subject to constraints
x + y ≥ 6, 2x + y ≥ 7, x + 4y ≥ 8, x ≥ 0, y ≥ 0
Solve the LPP graphically:
Minimize Z = 4x + 5y
Subject to the constraints 5x + y ≥ 10, x + y ≥ 6, x + 4y ≥ 12, x, y ≥ 0
Solution: Convert the constraints into equations and find the intercept made by each one of it.
| Inequations | Equations | X intercept | Y intercept | Region |
| 5x + y ≥ 10 | 5x + y = 10 | ( ___, 0) | (0, 10) | Away from origin |
| x + y ≥ 6 | x + y = 6 | (6, 0) | (0, ___ ) | Away from origin |
| x + 4y ≥ 12 | x + 4y = 12 | (12, 0) | (0, 3) | Away from origin |
| x, y ≥ 0 | x = 0, y = 0 | x = 0 | y = 0 | 1st quadrant |
∵ Origin has not satisfied the inequations.
∴ Solution of the inequations is away from origin.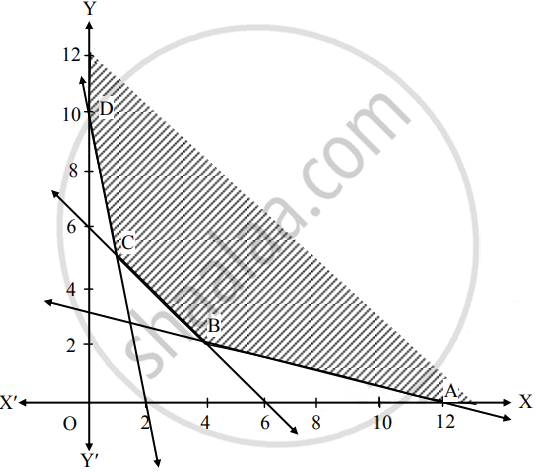
The feasible region is unbounded area which is satisfied by all constraints.
In the figure, ABCD represents
The set of the feasible solution where
A(12, 0), B( ___, ___ ), C ( ___, ___ ) and D(0, 10).
The coordinates of B are obtained by solving equations
x + 4y = 12 and x + y = 6
The coordinates of C are obtained by solving equations
5x + y = 10 and x + y = 6
Hence the optimum solution lies at the extreme points.
The optimal solution is in the following table:
| Point | Coordinates | Z = 4x + 5y | Values | Remark |
| A | (12, 0) | 4(12) + 5(0) | 48 | |
| B | ( ___, ___ ) | 4( ___) + 5(___ ) | ______ | ______ |
| C | ( ___, ___ ) | 4( ___) + 5(___ ) | ______ | |
| D | (0, 10) | 4(0) + 5(10) | 50 |
∴ Z is minimum at ___ ( ___, ___ ) with the value ___
