Advertisements
Advertisements
Question
The postmaster of a local post office wishes to hire extra helpers during the Deepawali season, because of a large increase in the volume of mail handling and delivery. Because of the limited office space and the budgetary conditions, the number of temporary helpers must not exceed 10. According to past experience, a man can handle 300 letters and 80 packages per day, on the average, and a woman can handle 400 letters and 50 packets per day. The postmaster believes that the daily volume of extra mail and packages will be no less than 3400 and 680 respectively. A man receives Rs 225 a day and a woman receives Rs 200 a day. How many men and women helpers should be hired to keep the pay-roll at a minimum ? Formulate an LPP and solve it graphically.
Solution
Let the postmaster hire x men and y women.
Clearly, x≥0, y≥0
Also, it is given that the number of temporary helpers must not exceed 10.
∴ x+y≤10
The given information can be represented in the tabular forms as
| Men (x) |
Women (y) |
Minimum Volume of Mails | |
| Letters | 300 | 400 | 3400 |
| Packages | 80 | 50 | 680 |
| Payroll (Rs) | 225 | 200 |
Thus, the given LPP can be stated mathematically as follows:
Minimise Z = 225x + 200y
Subject to the constraints:
x+y≤10 .....(1)
300x+400y≥3400 (constraint on letters)
⇒3x+4y≥34 .....(2)
80x+50y≥680 (constraint on packages)
8x+5y≥68 .....(3)
and x, y≥0 .....(4)
Converting the inequations into equations, we obtain the lines x + y = 10, 3x + 4y = 34, 8x + 5y = 68, x = 0 and y = 0.
These lines are drawn and the feasible region of the LPP is shaded. It is observed that the feasible region is a point (6, 4).
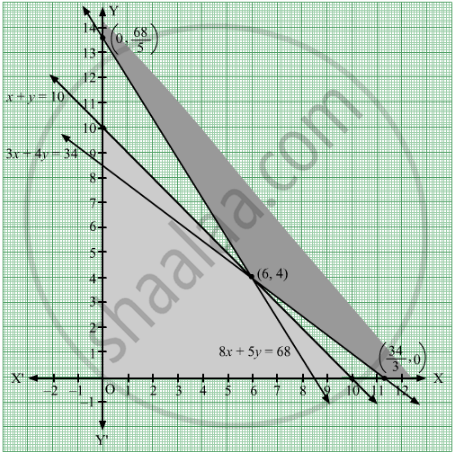
The value of the objective function at this point is given as Z = 2,150.
So, the minimum value of Z is 2150 at the point (6, 4).
Hence, the postmaster should hire 6 men and 4 women to keep the pay-roll at a minimum of Rs 2,150.
APPEARS IN
RELATED QUESTIONS
The corner points of the feasible region determined by the following system of linear inequalities:
2x + y ≤ 10, x + 3y ≤ 15, x, y ≥ 0 are (0, 0), (5, 0), (3, 4) and (0, 5). Let Z = px + qy, where p, q > 0. Condition on p and q so that the maximum of Z occurs at both (3, 4) and (0, 5) is
Solve the following L.P.P. by graphical method :
Minimize : Z = 7x + y subject to 5x + y ≥ 5, x + y ≥ 3, x ≥ 0, y ≥ 0.
Solve the following L.P.P. by graphical method:
Minimize: Z = 6x + 2y subject to x + 2y ≥ 3, x + 4y ≥ 4, 3x + y ≥ 3, x ≥ 0, y ≥ 0.
Choose the correct alternative :
The maximum value of z = 10x + 6y, subjected to the constraints 3x + y ≤ 12, 2x + 5y ≤ 34, x ≥ 0, y ≥ 0 is.
Fill in the blank :
The region represented by the in equations x ≤ 0, y ≤ 0 lines in _______ quadrants.
Graphical solution set of x ≤ 0, y ≥ 0 in xy system lies in second quadrant.
Solve the following problem :
A Company produces mixers and processors Profit on selling one mixer and one food processor is ₹ 2000 and ₹ 3000 respectively. Both the products are processed through three machines A, B, C The time required in hours by each product and total time available in hours per week on each machine are as follows:
| Machine/Product | Mixer per unit | Food processor per unit | Available time |
| A | 3 | 3 | 36 |
| B | 5 | 2 | 50 |
| C | 2 | 6 | 60 |
How many mixers and food processors should be produced to maximize the profit?
Solve the following problem :
A firm manufacturing two types of electrical items A and B, can make a profit of ₹ 20 per unit of A and ₹ 30 per unit of B. Both A and B make use of two essential components, a motor and a transformer. Each unit of A requires 3 motors and 2 transformers and each unit of B requires 2 motors and 4 transformers. The total supply of components per month is restricted to 210 motors and 300 transformers. How many units of A and B should be manufacture per month to maximize profit? How much is the maximum profit?
Choose the correct alternative:
If LPP has optimal solution at two point, then
Choose the correct alternative:
The maximum value of Z = 3x + 5y subjected to the constraints x + y ≤ 2, 4x + 3y ≤ 12, x ≥ 0, y ≥ 0 is
Choose the correct alternative:
The minimum value of Z = 4x + 5y subjected to the constraints x + y ≥ 6, 5x + y ≥ 10, x, y ≥ 0 is
Choose the correct alternative:
The corner points of the feasible region are (0, 3), (3, 0), (8, 0), `(12/5, 38/5)` and (0, 10), then the point of maximum Z = 6x + 4y = 48 is at
Choose the correct alternative:
The corner points of the feasible region are (4, 2), (5, 0), (4, 1) and (6, 0), then the point of minimum Z = 3.5x + 2y = 16 is at
State whether the following statement is True or False:
The maximum value of Z = 5x + 3y subjected to constraints 3x + y ≤ 12, 2x + 3y ≤ 18, 0 ≤ x, y is 20
State whether the following statement is True or False:
If LPP has two optimal solutions, then the LPP has infinitely many solutions
State whether the following statement is True or False:
If the corner points of the feasible region are `(0, 7/3)`, (2, 1), (3, 0) and (0, 0), then the maximum value of Z = 4x + 5y is 12
State whether the following statement is True or False:
Corner point method is most suitable method for solving the LPP graphically
A set of values of variables satisfying all the constraints of LPP is known as ______
The feasible region represented by the inequations x ≥ 0, y ≤ 0 lies in ______ quadrant.
If the feasible region is bounded by the inequations 2x + 3y ≤ 12, 2x + y ≤ 8, 0 ≤ x, 0 ≤ y, then point (5, 4) is a ______ of the feasible region
Maximize Z = 2x + 3y subject to constraints
x + 4y ≤ 8, 3x + 2y ≤ 14, x ≥ 0, y ≥ 0.
Maximize Z = 5x + 10y subject to constraints
x + 2y ≤ 10, 3x + y ≤ 12, x ≥ 0, y ≥ 0
Amartya wants to invest ₹ 45,000 in Indira Vikas Patra (IVP) and in Public Provident fund (PPF). He wants to invest at least ₹ 10,000 in PPF and at least ₹ 5000 in IVP. If the rate of interest on PPF is 8% per annum and that on IVP is 7% per annum. Formulate the above problem as LPP to determine maximum yearly income.
Solution: Let x be the amount (in ₹) invested in IVP and y be the amount (in ₹) invested in PPF.
x ≥ 0, y ≥ 0
As per the given condition, x + y ______ 45000
He wants to invest at least ₹ 10,000 in PPF.
∴ y ______ 10000
Amartya wants to invest at least ₹ 5000 in IVP.
∴ x ______ 5000
Total interest (Z) = ______
The formulated LPP is
Maximize Z = ______ subject to
______
Solve the LPP graphically:
Minimize Z = 4x + 5y
Subject to the constraints 5x + y ≥ 10, x + y ≥ 6, x + 4y ≥ 12, x, y ≥ 0
Solution: Convert the constraints into equations and find the intercept made by each one of it.
| Inequations | Equations | X intercept | Y intercept | Region |
| 5x + y ≥ 10 | 5x + y = 10 | ( ___, 0) | (0, 10) | Away from origin |
| x + y ≥ 6 | x + y = 6 | (6, 0) | (0, ___ ) | Away from origin |
| x + 4y ≥ 12 | x + 4y = 12 | (12, 0) | (0, 3) | Away from origin |
| x, y ≥ 0 | x = 0, y = 0 | x = 0 | y = 0 | 1st quadrant |
∵ Origin has not satisfied the inequations.
∴ Solution of the inequations is away from origin.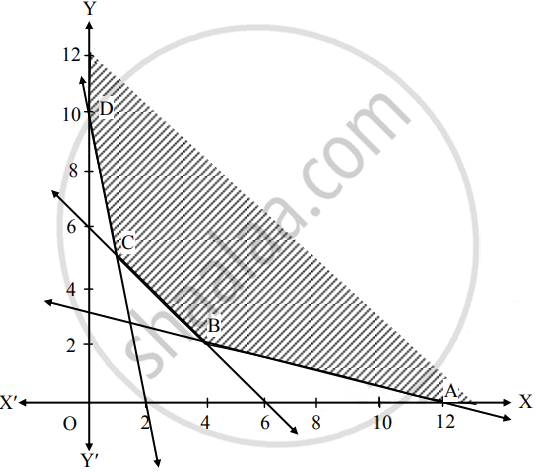
The feasible region is unbounded area which is satisfied by all constraints.
In the figure, ABCD represents
The set of the feasible solution where
A(12, 0), B( ___, ___ ), C ( ___, ___ ) and D(0, 10).
The coordinates of B are obtained by solving equations
x + 4y = 12 and x + y = 6
The coordinates of C are obtained by solving equations
5x + y = 10 and x + y = 6
Hence the optimum solution lies at the extreme points.
The optimal solution is in the following table:
| Point | Coordinates | Z = 4x + 5y | Values | Remark |
| A | (12, 0) | 4(12) + 5(0) | 48 | |
| B | ( ___, ___ ) | 4( ___) + 5(___ ) | ______ | ______ |
| C | ( ___, ___ ) | 4( ___) + 5(___ ) | ______ | |
| D | (0, 10) | 4(0) + 5(10) | 50 |
∴ Z is minimum at ___ ( ___, ___ ) with the value ___
If z = 200x + 500y .....(i)
Subject to the constraints:
x + 2y ≥ 10 .......(ii)
3x + 4y ≤ 24 ......(iii)
x, 0, y ≥ 0 ......(iv)
At which point minimum value of Z is attained.
Graphical solution set of the inequations x ≥ 0 and y ≤ 0 lies in ______ quadrant.
