Advertisements
Advertisements
Question
Suppose you drop a die at random on the rectangular region shown in the given figure. What is the probability that it will land inside the circle with diameter 1m?
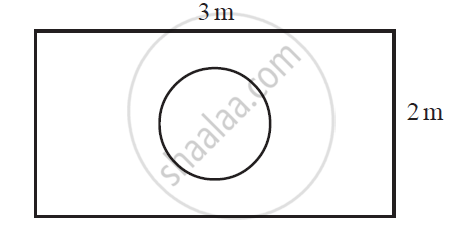
Solution
Area of rectangle = l × b = 3 × 2 = 6 m2
Area of circle (of diameter 1 m) = `pir^2= pi((1)/2)^2 m^2= pi/4 m^2`
∴ Probability that the die will fall inside the
Cicle = `"Area of the favourable region"/ "Area of the whole region"`
= `"Area of the circle"/"Area of the rectangle"`
`= (pi/4)/6 = pi/4 xx 1/6 = pi/24`
P (die will land inside the circle) = `(pi/4)/6= pi/24`
APPEARS IN
RELATED QUESTIONS
Following experiment have equally likely outcomes? Explain.
A driver attempts to start a car. The car starts or does not start.
If P(E) = 0.05, what is the probability of ‘not E’?
A box contains 12 balls out of which x are black. If one ball is drawn at random from the box, what is the probability that it will be a black ball?
If 6 more black balls are put in the box, the probability of drawing a black ball is now double of what it was before. Find x.
A bag contains 4 red, 5 black and 6 white balls. A ball is drawn from the bag at random. Find the probability that the ball drawn is Red or white
What is the probability that a number selected at random from the number 1,2,2,3,3,3, 4, 4, 4, 4 will be their average?
A bag contains 8 red, 6 white and 4 black balls. A ball is drawn at random from the bag. Find the probability that the drawn ball is Neither white nor black
A number is selected at random from first 50 natural numbers. Find the probability that it is a multiple of 3 and 4.
A bag contains 100 identical marble stones which are numbered 1 to 100. If one stone is drawn at random from the bag, find the probability that it bears a perfect square number.
A circle with diameter 20 cm is drawn somewhere on a rectangular piece of paper with length 40 cm and width 30 cm. This paper is kept horizontal on table top and a dice, very small in size, is dropped on the rectangular paper without seeing towards it. If the dice falls and lands on the paper only, find the probability that it will fall and land inside the circle.
Two dice (each bearing numbers 1 to 6) are rolled together. Find the probability that the sum of the numbers on the upper-most faces of two dice is 4 or 5.
Offices in Delhi are open for five days in a week (Monday to Friday). Two employees of an office remain absent for one day in the same particular week. Find the probability that they remain absent on different days.
Six faces of a die are as shown below.
If the die is rolled once, find the probability of -‘D’ appears on upper face.
Namita tossed a coin once. What is the probability of getting tail?
A die has 6 faces marked by the given numbers as shown below:
| 1 | 2 | 3 | – 1 | – 2 | – 3 |
The die is thrown once. What is the probability of getting the smallest integer?
If P(A) denotes the probability of an event A, then ______.
The probability of getting a bad egg in a lot of 400 is 0.035. The number of bad eggs in the lot is ______.
A student says that if you throw a die, it will show up 1 or not 1. Therefore, the probability of getting 1 and the probability of getting ‘not 1’ each is equal to `1/2`. Is this correct? Give reasons.
A bag contains 30 discs numbered from 1 to 30. One disc is drawn at random from the bag. Find the probability that it bears a number greater than 25.
For an event E, if `P(E) + P(barE)` = q, then the value of q2 − 4 is ______.
