Advertisements
Advertisements
Question
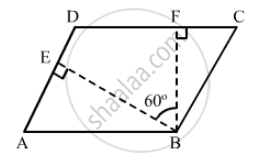
The angle between the altitudes of a parallelogram, through the same vertex of an obtuse angle of the parallelogram is 60°. Find the angles of the parallelogram.
Solution
\[\text{ Draw a parallelogram ABCD } . \]
\[\text{ Drop a perpendicular from B to the side AD, at the point E } . \]
\[\text{ Drop perpendicular from B to the side CD, at the point F } . \]
\[\text{ In the quadrilateral BEDF }: \]
\[\angle EBF = 60°, \angle BED = 90°\]
\[\angle BFD = 90°e \]
\[\angle EDF = 360° - (60° + 90° + 90°) = 120°\]
\[\text{ In a parallelogram, opposite angles are congruent and adjacent angles are supplementary } . \]
\[\text{ In the parallelogram ABCD }: \]
\[\angle B = \angle D = 120° \]
\[\angle A = \angle C = 180°e - 120° = 60 °\]
APPEARS IN
RELATED QUESTIONS
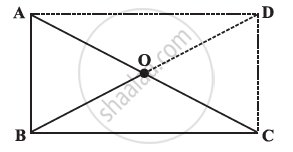
ABC is a right-angled triangle and O is the mid point of the side opposite to the right angle. Explain why O is equidistant from A, B and C. (The dotted lines are drawn additionally to help you)
Which of the following statement is true for a rectangle?
It has two pairs of equal sides.
Which of the following statement is true for a square?
It is a rectangle.
Find the length of the diagonal of a rectangle whose sides are 12 cm and 5 cm.
Draw a square whose each side measures 4.8 cm.
State with Reason Whether the Following Statement is ‘True’ Or ‘False’.
Every rectangle is a parallelogram.
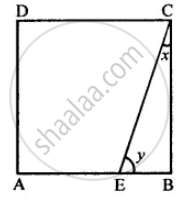
The following figure is a rectangle in which x: y = 3: 7; find the values of x and y.
For which of the following figures, diagonals are equal?
Every trapezium is a rectangle.
A rectangular MORE is shown below:
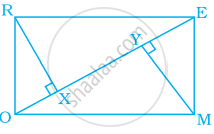
Answer the following questions by giving appropriate reason.
- Is RE = OM?
- Is ∠MYO = ∠RXE?
- Is ∠MOY = ∠REX?
- Is ΔMYO ≅ ΔRXE?
- Is MY = RX?
