Advertisements
Advertisements
Question
The area of the square that can be inscribed in a circle of radius 8 cm is ______.
Options
256 cm2
128 cm2
`64sqrt(2)` cm2
64 cm2
Solution
The area of the square that can be inscribed in a circle of radius 8 cm is 128 cm2.
Explanation:
Given, radius of circle, r = OC = 8 cm.
∴ Diameter of the circle = AC
= 2 × OC
= 2 × 8
= 16 cm
Which is equal to the diagonal of a square.
Let side of square be x.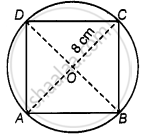
In right-angled ΔABC,
AC2 = AB2 + BC2 ...[By Pythagoras theorem]
⇒ (16)2 = x2 + x2
⇒ 256 = 2x2
⇒ x2 = 128
∴ Area of square = x2 = 128 cm2
APPEARS IN
RELATED QUESTIONS
In the given figure, OACB is a quadrant of circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the
(i) Quadrant OACB
(ii) Shaded region
[Use Π = 22/7]

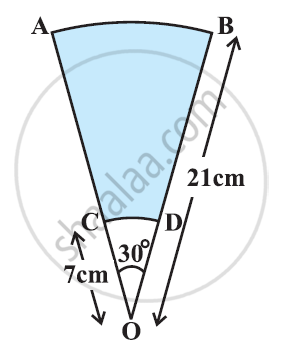
AB and CD are respectively arcs of two concentric circles of radii 21 cm and 7 cm and centre O (see the given figure). If ∠AOB = 30°, find the area of the shaded region. [Use Π = 22/7]
In the given figure, ABC is a quadrant of a circle of radius 14 cm and a semicircle is drawn with BC as diameter. Find the area of the shaded region. [Use Π = 22/7]

Calculate the area of the designed region in the given figure common between the two quadrants of circles of radius 8 cm each. [Use Π = 22/7]

Find the areas of the triangle whose sides are 42 cm, 34 cm and 20 cm. Also, find the height corresponding to the longest side.
The perimeter of a triangular field is 240m, and its sides are in the ratio 25:17:12. Find the area of the field. Also, find the cost of ploughing the field at ₹ 40 per `m^2`
If the area of an equilateral triangle is `36sqrt3 cm^2` find its perimeter.
In Figure 5, ABCD is a quadrant of a circle of radius 28 cm and a semi circle BEC is drawn with BC as diameter. Find the area of the shaded region ?\[[Use\pi = \frac{22}{7}]\]
A plane left 30 minutes later than the scheduled time and in order to reach its destination 1500 km away on time, it has to increase its speed by 250 km/hr from its usual speed. Find the usual speed of the plane.
Find the area of the flower bed (with semi-circular ends) shown in figure.