Advertisements
Advertisements
Question
The base of a right – angled triangle measures 48 cm and its hypotenuse measures 50 cm. Find the area of the triangle.
Solution
Base = 48 cm
Hypotenuse 50 cm
First we will find the height of the triangle; let the height be ‘p’.
⇒ `(Hypotenuse)^2=(base)^2+p^2`
⇒`50^2=48^2+p^2`
⇒ `P^2=50^2-48^2`
⇒`P^2=(50-48) (50+48)`
⇒`P^2=2xx98`
⇒`P^2=196`
⇒`P=14cm`
Area of the triangle=`1/2xxbasexxheight`
=`1/2xx48xx14`
=`336cm^2`
APPEARS IN
RELATED QUESTIONS
An arc of a circle is of length 5π cm and the sector it bounds has an area of 20 π cm2. Find the radius of the circle.
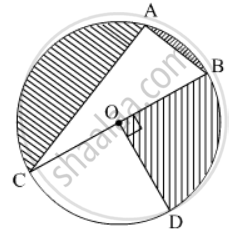
In the given figure, O is the centre of the circle with AC = 24 cm, AB = 7 cm and ∠BOD = 90°. Find the area of the shaded region.
A gardener wants to fence a circular garden of diameter 21 m. Find the length of the rope he needs to purchase, if he makes 2 rounds of fence. Also find the costs of the rope, if it cost ₹ 4 per meter. (Take `pi = 22/7`)
The perimeter of the quadrant of a circle is 25 cm. Find its area.
The radius of a circular garden is 100 m. There is a road 10 m wide, running all around it. Find the area of the road and the cost of levelling it at Rs 20 per m2. [Use π = 3.14]
The area of an equilateral triangle is `49sqrt(3)` cm2 . Taking each angular point as centre, circles are drawn with radius equal to half the length of the side of the triangle. Find the area of the triangle not included in the circles. [Take π = 1.73]
Sand from a cylindrical bucket 32 cm in height and 18 cm in radius is poured onto the ground making a conical heap 24 cm high. Find the radius of the conical heap.
Construct an angle PQR = 45°. Mark a point S on QR such that QS = 4.5 cm. Construct a circle to touch PQ at Q and also to pass through S.
Complete the table below.
| Radius (r) | Diameter (d) | Circumference (c) |
| ...... | ...... | 616 cm |
The circumference of a circle is numerically equal to its area. Find the area and circumference of the circle.
