Advertisements
Advertisements
Question
The area of an equilateral triangle is `49sqrt(3)` cm2 . Taking each angular point as centre, circles are drawn with radius equal to half the length of the side of the triangle. Find the area of the triangle not included in the circles. [Take π = 1.73]
Solution
Area of equilateral triangle`= 49 sqrt(3)`
`=> sqrt(3)/4xx("Side")^2 = 49sqrt(3)`
⇒ (Side)2 = 72 × 22
⇒ Side = 14 cm
Radius of the circle = Half of the side of the triangle = 7 cm
Area of triangle not included in the circle = Area of triangle − Area of 3 sectors having central angle 60∘
`=49sqrt(3) - 3xx60^circ/360^circxx22/7xx7xx7`
= 84.77 - 77
= 7.77 cm2
Hence, the required area is 7.77 cm2
APPEARS IN
RELATED QUESTIONS
The radius of a circle is 7 cm. find the circumference of the circle.
The circumference of a circle exceeds the diameter by 16.8 cm. Find the radius of the circle.
Find the area of the sector of a circle whose radius is 14 cm and angle of sector is 45º
A steel wire when bent is the form of square encloses an area of 12 cm2. If the same wire is bent in form of circle. Find the area of circle.
A square water tank has its side equal to 40m, there are 4 semicircular flower beds grassy plots all around it. Find the cost of turfing the plot at Rs 1.25/sq.m
Choose the correct alternative answer for the following question.
The radius of a circle is 5 m. Find the circumference of the circle whose area is 49 times the area of the given circle.
Find the area of a circle whose circumference is 22 cm.
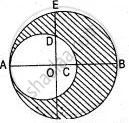
In the adjoining figure, the crescent is formed by two circles which touch at the point A. O is the centre of bigger circle. If CB = 9 cm and DE = 5 cm, find the area of the shaded portion.
A bucket is raised from a well by means of a rope wound round a wheel of diameter 35 cm. If the bucket ascends in 2 minutes with a uniform speed of 1.1 m per sec, calculate the number of complete revolutions the wheel makes in raising the bucket.
