Advertisements
Advertisements
Question
The bob of a pendulum at rest is given a sharp hit to impart a horizontal velocity \[\sqrt{10 \text{ gl }}\], where l is the length of the pendulum. Find the tension in the string when (a) the string is horizontal, (b) the bob is at its highest point and (c) the string makes an angle of 60° with the upward vertical.
Solution
(a) Let the velocity at B be \[\text{v}_1\] .
\[\frac{1}{2}\text{ m}\nu^2 = \frac{1}{2}\text{m} v_1^2 + \text{mgl}\]
\[ \Rightarrow \frac{1}{2}\text{m} \left( 10 \text{gl} \right) = \frac{1}{2}\text{m} \nu_1^2 + \text{mgl}\]
\[ \nu_1^2 = 8 \text{gl}\]

So, the tension in the string at the horizontal position,
\[\text{ T }= \frac{\text{ m} \nu^2}{\text{ R}} = \text{ m }\frac{8 \text{ gl} }{\text{l}}\]
\[ = 8 \text{mg}\]
(b) Let the velocity at C be \[\text{v}_2\] .
\[\frac{1}{2}\text{ m }\nu^2 = \frac{1}{2}\text{ m }\nu_2^2 + \text{ mg (2l)}\]
\[\Rightarrow \frac{1}{2}\text{m} 10 \text{ gl } = \frac{1}{2}\text{ m }\nu_2^2 + 2\text{ mgl }\]
\[ \Rightarrow \nu_2^2 = 6 \text{ gl }\]
So, the tension in the string is given by \[T_C = \frac{\text{mv}_2^2}{\text{l}} - \text{mg = 5 mg}\]
(c) Let the velocity at point D be \[\nu_4\] .
Again,
\[\frac{1}{2}\text{m} \nu^2 = \frac{1}{2}\text{m} \nu_3^2 + \text{mgl} \left( 1 + \cos 60^\circ\right)\]
\[ \Rightarrow \nu_3^2 = 7 \text{gl}\]
So, the tension in the string,
\[\text{T}_\text{D} = \frac{\text{m} \nu_3^2}{\text{l}} - \text{mg} \cos 60^\circ\]
\[ = \text{m}\frac{\left( 7 \text{gl} \right)}{\text{l}} - 0 . 5 \text{mg}\]
\[ = 7 \text{ mg - 0 . 5 mg }\]
\[ = 6 . 5 \text{ mg}\]
APPEARS IN
RELATED QUESTIONS
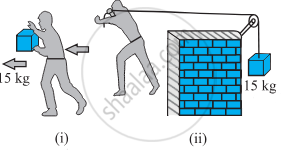
In Figure (i) the man walks 2 m carrying a mass of 15 kg on his hands. In Figure (ii), he walks the same distance pulling the rope behind him. The rope goes over a pulley, and a mass of 15 kg hangs at its other end. In which case is the work done greater?
A ball is given a speed v on a rough horizontal surface. The ball travels through a distance l on the surface and stops. what are the initial and final kinetic energies of the ball?
A ball is given a speed v on a rough horizontal surface. The ball travels through a distance l on the surface and stops. What is the work done by the kinetic friction?
The US athlete Florence Griffith-Joyner won the 100 m sprint gold medal at Seoul Olympics in 1988, setting a new Olympic record of 10⋅54 s. Assume that she achieved her maximum speed in a very short time and then ran the race with that speed till she crossed the line. Take her mass to be 50 kg. Assuming that the track, wind etc. offered an average resistance of one-tenth of her weight, calculate the work done by the resistance during the run.
The US athlete Florence Griffith-Joyner won the 100 m sprint gold medal at Seoul Olympics in 1988, setting a new Olympic record of 10⋅54 s. Assume that she achieved her maximum speed in a very short time and then ran the race with that speed till she crossed the line. Take her mass to be 50 kg. What power Griffith-Joyner had to exert to maintain uniform speed?
An unruly demonstrator lifts a stone of mass 200 g from the ground and throws it at his opponent. At the time of projection, the stone is 150 cm above the ground and has a speed of 3 m/s. Calculate the work done by the demonstrator during the process. If it takes one second for the demonstrator to lift the stone and throw it, what horsepower does he use?
A block of mass 5 kg is suspended from the end of a vertical spring which is stretched by 10 cm under the load of the block. The block is given a sharp impulse from below, so that it acquires an upward speed of 2 m/s. How high will it rise? Take g = 10 m/s2.
A simple pendulum consists of a 50 cm long string connected to a 100 g ball. The ball is pulled aside so that the string makes an angle of 37° with the vertical and is then released. Find the tension in the string when the bob is at its lowest position.
A heavy particle is suspended by a 1⋅5 m long string. It is given a horizontal velocity of \[\sqrt{57} \text{m/s}\] (a) Find the angle made by the string with the upward vertical when it becomes slack. (b) Find the speed of the particle at this instant. (c) Find the maximum height reached by the particle over the point of suspension. Take g = 10 m/s2.
A simple pendulum of length L with a bob of mass m is deflected from its rest position by an angle θ and released (following figure). The string hits a peg which is fixed at a distance x below the point of suspension and the bob starts going in a circle centred at the peg. (a) Assuming that initially the bob has a height less than the peg, show that the maximum height reached by the bob equals its initial height. (b) If the pendulum is released with \[\theta = 90^\circ \text{ and x = L}/2\] , find the maximum height reached by the bob above its lowest position before the string becomes slack. (c) Find the minimum value of x/L for which the bob goes in a complete circle about the peg when the pendulum is released from \[\theta = 90^\circ \]

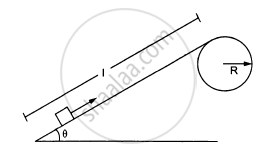
Figure ( following ) shows a smooth track which consists of a straight inclined part of length l joining smoothly with the circular part. A particle of mass m is projected up the incline from its bottom. Find the minimum projection-speed \[\nu_0\] for which the particle reaches the top of the track.
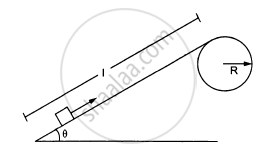
Figure ( following ) shows a smooth track which consists of a straight inclined part of length l joining smoothly with the circular part. A particle of mass m is projected up the incline from its bottom.Assuming that the projection-speed is only slightly greater than \[\nu_0\] , where will the block lose contact with the track?
A chain of length l and mass m lies on the surface of a smooth sphere of radius R > l with one end tied to the top of the sphere. Find the tangential acceleration \[\frac{d\nu}{dt}\] of the chain when the chain starts sliding down.
An electron and a proton are moving under the influence of mutual forces. In calculating the change in the kinetic energy of the system during motion, one ignores the magnetic force of one on another. This is because ______.
Two bodies of unequal mass are moving in the same direction with equal kinetic energy. The two bodies are brought to rest by applying retarding force of same magnitude. How would the distance moved by them before coming to rest compare?
A raindrop of mass 1.00 g falling from a height of 1 km hits the ground with a speed of 50 ms–1. Calculate
- the loss of P.E. of the drop.
- the gain in K.E. of the drop.
- Is the gain in K.E. equal to a loss of P.E.? If not why.
Take g = 10 ms–2
