Advertisements
Advertisements
Question
A heavy particle is suspended by a 1⋅5 m long string. It is given a horizontal velocity of \[\sqrt{57} \text{m/s}\] (a) Find the angle made by the string with the upward vertical when it becomes slack. (b) Find the speed of the particle at this instant. (c) Find the maximum height reached by the particle over the point of suspension. Take g = 10 m/s2.
Solution
\[\text{ Given }: \]
\[\text{ Length of the string, L = 1 . 5 m}\]
\[\text{ Initial speed of the particle, u } = \sqrt{57} \text{ m/s }\]
\[(\text{a}) \text{ mg } \cos \theta = \frac{\text{m}\nu^2}{\text{L}}\]
\[ \nu^2 = \text{Lg} \cos \theta . . . (\text{i})\]
Change in K.E. = Work done
\[\frac{1}{2}\text{m}\nu^2 - \frac{1}{2}\text{mu}^2 = - \text{mgh}\]
\[ \Rightarrow \nu^2 - 57 = - 2 \times 1 . 5 \text{g} \left( 1 + \cos \theta \right) \]
\[ \Rightarrow \nu^2 = 57 - 3\text{g }\left( 1 + \cos \theta \right) . . . (\text{ii})\]

Putting the value of \[\nu\] from equation (i),
\[15 \cos \theta = 57 - 3g \left( 1 + \cos \theta \right)\]
\[ \Rightarrow 15 \cos \theta = 57 - 30 - 30 \cos \theta\]
\[ \Rightarrow 45 \theta = 27\]
\[ \Rightarrow \cos \theta = \frac{3}{5}\]
\[ \Rightarrow \theta = \cos^{- 1} \frac{3}{5} = 53^\circ\]
(b) From equation (ii),
\[\nu = \sqrt{57 - 3g \left( 1 + \cos \theta \right)}\]
\[ = \sqrt{9} = 3 \text{ m/s}\]
\[ = 1 . 5 \cos \theta + \frac{\text{u}^2 \sin^2 \theta}{2 \text{g}}\]
\[ = \left( 1 . 5 \right) \times \frac{3}{5} + \frac{9 \times \left( 0 . 8 \right)^2}{2 \times 10}\]
\[ = 1 . 2 \text{m}\]
APPEARS IN
RELATED QUESTIONS
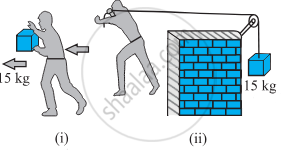
In Figure (i) the man walks 2 m carrying a mass of 15 kg on his hands. In Figure (ii), he walks the same distance pulling the rope behind him. The rope goes over a pulley, and a mass of 15 kg hangs at its other end. In which case is the work done greater?
Is work-energy theorem valid in non-inertial frames?
A ball is given a speed v on a rough horizontal surface. The ball travels through a distance l on the surface and stops. what are the initial and final kinetic energies of the ball?
Consider the situation of the previous question from a frame moving with a speed v0 parallel to the initial velocity of the block. (a) What are the initial and final kinetic energies? (b) What is the work done by the kinetic friction?
A water pump lifts water from 10 m below the ground. Water is pumped at a rate of 30 kg/minute with negligible velocity. Calculate the minimum horsepower that the engine should have to do this.
An unruly demonstrator lifts a stone of mass 200 g from the ground and throws it at his opponent. At the time of projection, the stone is 150 cm above the ground and has a speed of 3 m/s. Calculate the work done by the demonstrator during the process. If it takes one second for the demonstrator to lift the stone and throw it, what horsepower does he use?
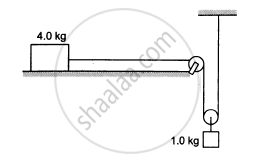
Consider the situation shown in the following figure. The system is released from rest and the block of mass 1 kg is found to have a speed 0⋅3 m/s after it has descended a distance of 1 m. Find the coefficient of kinetic friction between the block and the table.
The bob of a stationary pendulum is given a sharp hit to impart it a horizontal speed of \[\sqrt{3 gl}\] . Find the angle rotated by the string before it becomes slack.
A chain of length l and mass m lies on the surface of a smooth sphere of radius R > l with one end tied to the top of the sphere. Find the gravitational potential energy of the chain with reference level at the centre of the sphere.
A chain of length l and mass m lies on the surface of a smooth sphere of radius R > l with one end tied to the top of the sphere. Suppose the chain is released and slides down the sphere. Find the kinetic energy of the chain, when it has slid through an angle θ.
A smooth sphere of radius R is made to translate in a straight line with a constant acceleration a. A particle kept on the top of the sphere is released at zero velocity with respect to the sphere. Find the speed of the particle with respect to the sphere as a function of the angle θ it slides.
An electron and a proton are moving under the influence of mutual forces. In calculating the change in the kinetic energy of the system during motion, one ignores the magnetic force of one on another. This is because ______.
A bullet of mass m fired at 30° to the horizontal leaves the barrel of the gun with a velocity v. The bullet hits a soft target at a height h above the ground while it is moving downward and emerges out with half the kinetic energy it had before hitting the target.
Which of the following statements are correct in respect of bullet after it emerges out of the target?
- The velocity of the bullet will be reduced to half its initial value.
- The velocity of the bullet will be more than half of its earlier velocity.
- The bullet will continue to move along the same parabolic path.
- The bullet will move in a different parabolic path.
- The bullet will fall vertically downward after hitting the target.
- The internal energy of the particles of the target will increase.
Give example of a situation in which an applied force does not result in a change in kinetic energy.
A raindrop of mass 1.00 g falling from a height of 1 km hits the ground with a speed of 50 ms–1. Calculate
- the loss of P.E. of the drop.
- the gain in K.E. of the drop.
- Is the gain in K.E. equal to a loss of P.E.? If not why.
Take g = 10 ms–2
A particle moves in one dimension from rest under the influence of a force that varies with the distance travelled by the particle as shown in the figure. The kinetic energy of the particle after it has travelled 3 m is ______.

