Advertisements
Advertisements
Question
The diagonals AC and BD of a quadrilateral ABCD intersect at right angles. Prove that the quadrilateral formed by joining the midpoints of quadrilateral ABCD is a rectangle.
Solution
The figure is as below:
Let ABCD be a quadrilateral where p, Q, R, S are the midpoint of sides AB, BC, CD, DA respectively. Diagonals AC and BD intersect at right angles at point O. We need to show that PQRS is a rectangle
Proof:
In ΔABC and ΔADC,
2PQ = AC and PQ || AC ....(1)
2RS = AC and RS || AC ....(2)
From (1) and (2), we get
PQ = RS and PQ || RS
Similarly, we can show that
PS = RQ and PS || RQ
Therefore, PQRS is a parallelogram.
Now, PQ || AC,
∴ ∠AOD = ∠PXO = 90° ....(Corresponding angles)
Again, BD || RQ,
∴ ∠PXO = ∠RQX = 90° ....(Corresponding angles)
Similarly, ∠QRS = ∠RSP = SPQ = 90°
Therefore, PQRD is a rectangle.
APPEARS IN
RELATED QUESTIONS
ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
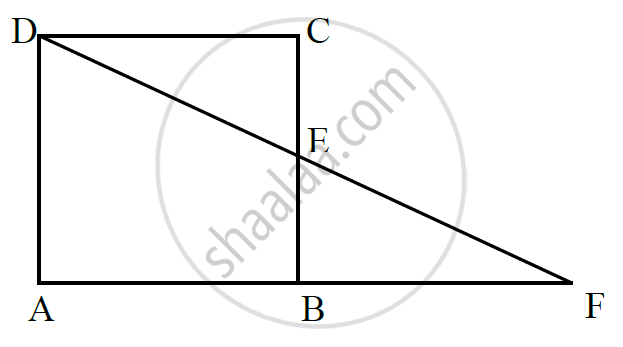
In below fig. ABCD is a parallelogram and E is the mid-point of side B If DE and AB when produced meet at F, prove that AF = 2AB.
In below Fig, ABCD is a parallelogram in which P is the mid-point of DC and Q is a point on AC such that CQ = `1/4` AC. If PQ produced meets BC at R, prove that R is a mid-point of BC.

BM and CN are perpendiculars to a line passing through the vertex A of a triangle ABC. If
L is the mid-point of BC, prove that LM = LN.
In parallelogram PQRS, L is mid-point of side SR and SN is drawn parallel to LQ which meets RQ produced at N and cuts side PQ at M. Prove that M is the mid-point of PQ.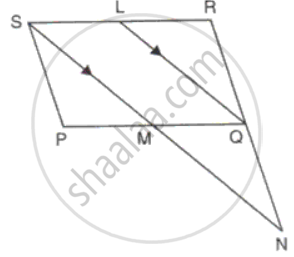
The diagonals of a quadrilateral intersect each other at right angle. Prove that the figure obtained by joining the mid-points of the adjacent sides of the quadrilateral is a rectangle.
In ΔABC, P is the mid-point of BC. A line through P and parallel to CA meets AB at point Q, and a line through Q and parallel to BC meets median AP at point R. Prove that: BC = 4QR
In ΔABC, D, E and F are the midpoints of AB, BC and AC.
Show that AE and DF bisect each other.
In AABC, D and E are two points on the side AB such that AD = DE = EB. Through D and E, lines are drawn parallel to BC which meet the side AC at points F and G respectively. Through F and G, lines are drawn parallel to AB which meet the side BC at points M and N respectively. Prove that BM = MN = NC.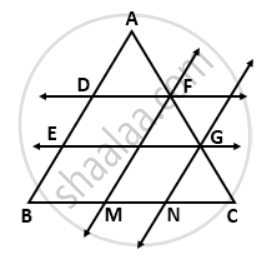
In ΔABC, D and E are the midpoints of the sides AB and AC respectively. F is any point on the side BC. If DE intersects AF at P show that DP = PE.
