Advertisements
Advertisements
Question
The diameters of front and rear wheels of a tractor are 80 cm and 2 m respectively. Find the number of revolutions that rear wheel will make in covering a distance in which the front wheel makes 1400 revolutions.
Solution
According to the question,
Diameter of front wheels = d1 = 80 cm
Diameter of rear wheels = d2 = 2 m = 200 cm
Let r1 be the radius of the front wheels = `80/2` = 40 cm
Let r2 be the radius of the rear wheels = `200/2` = 100 cm
Now, Circumference of the front wheels = 2πr
= `2 xx 22/7 xx 40`
= `1760/7` cm
Circumference of the rear wheels = 2πr
= `2 xx 22/7 × 100`
= `4400/7` cm
No. of revolutions made by the front wheel = 1400
∴ Distance covered by the front wheel
= `1400 xx 1760/7`
= 352000 cm
Number of revolutions made by rear wheel in covering a distance in which the front wheel makes 1400 revolutions
= `"Distance covered by front wheel"/"Circumference of the rear wheel"`
= `352000/(4400/7)`
= `(352000 xx 7)/4400`
= 560 revolutions.
APPEARS IN
RELATED QUESTIONS
A sector is cut from a circle of radius 21 cm. The angle of the sector is 150º. Find the length of its arc and area.
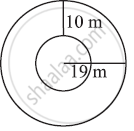
Find the circumference of the inner and the outer circles, shown in the adjoining figure? (Take π = 3.14)
The area of circle, inscribed in equilateral triangle is 154 cms2. Find the perimeter of
triangle.
Choose the correct alternative answer for the following question.
Two circular pieces of equal radii and maximum area, touching each other are cut out from a rectangular cardboard of dimensions 14 cm ⨯ 7 cm. Find the area of the remaining cardboard.
The area of a circle is 49 π cm2. Its circumference is:
A circus tent is cylindrical to a height of 5 m and conical above it. If its diameter is 42 m and slant height of the cone is 53 m, calculate the total area of the canvas required.
Sand from a cylindrical bucket 32 cm in height and 18 cm in radius is poured onto the ground making a conical heap 24 cm high. Find the radius of the conical heap.
Complete the table below.
| Radius (r) | Diameter (d) | Circumference (c) |
| ...... | ...... | 616 cm |
In covering a distance s metres, a circular wheel of radius r metres makes `s/(2πr)` revolutions. Is this statement true? Why?
