Advertisements
Advertisements
Question
The given figure shows a trapezium in which AB is parallel to DC and diagonals AC and BD intersect at point P. If AP : CP = 3 : 5,

Find:
- ∆APB : ∆CPB
- ∆DPC : ∆APB
- ∆ADP : ∆APB
- ∆APB : ∆ADB
Solution
i. Since ΔAPB and ΔCPB have common vertex at B and their bases AP and PC are along the same straight line
`∴(ar(ΔAPB))/(ar(ΔCPB)) = (AP)/(PC) = 3/5`
ii. Since ΔDPC and ΔBPA are similar
∴ `(ar(Δ DPC))/(ar(ΔCPB)) = ((PC)/(AP))^2 = (5/3)^2 = 25/9`
iii. Since ΔADP and ΔAPB have common vertex at A and their bases DP and PB are along the same straight line
∴ `(ar (ΔADP))/(ar(Δ APB)) = (DP)/(PB) = 5/3`
`(ΔAPB ∼ ΔCPD ⇒ (AP)/(PC) = (BP)/(PD) = 3/5)`
iv. Since ΔAPB and ΔADB have common vertex at A and their bases BP and BD are along the same straight line.
∴ `(ar(ΔAPB))/(ar(ΔADB)) = (PB)/(BD) = 3/8`
`(ΔAPB ∼ ΔCPD => (AP)/(PC) = (BP)/(PD) = 3/5 => (BP)/(BD) = 3/8)`
APPEARS IN
RELATED QUESTIONS
Prove that the angle bisector of a triangle divides the side opposite to the angle in the ratio of the remaining sides.
ABCD is a quadrilateral in which AD = BC. If P, Q, R, S be the midpoints of AB, AC, CD and BD respectively, show that PQRS is a rhombus.

ΔABC is right angled at A and AD⊥BC. If BC = 13cm and AC = 5cm, find the ratio of the areas of ΔABC and ΔADC.
In ∆ABC and ∆DEF ∠B = ∠E, ∠F = ∠C and AB = 3DE then which of the statements regarding the two triangles is true ?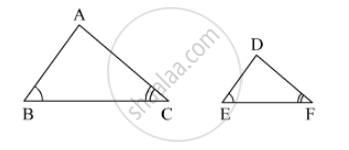
In the following figure, point D divides AB in the ratio 3 : 5. Find :
DE = 2.4 cm, find the length of BC.

In ΔABC, D and E are the mid-point on AB and AC such that DE || BC.
If AD = 4, AE = 8, DB = x - 4 and EC = 3x - 19, find x.
Find the scale factor in each of the following and state the type of size transformation:
Model area = 75cm2, Actual area = 3cm2
A map is drawn to scale of 1:20000. Find: The distance on the map representing 4km
State whether the following triangles are similar or not: If yes, then write the test of similarity.
∠P = 35°, ∠X = 35° and ∠Q = 60°, ∠Y = 60°

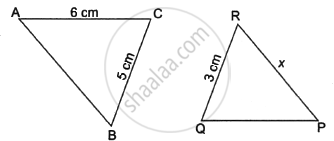
In the given figure, ΔABC ∼ ΔQPR, If AC = 6 cm, BC = 5 cm, QR = 3 cm and PR = x; them the value of x is ______.