Advertisements
Advertisements
Question
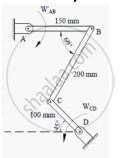
The link CD of the mechanism shown in Figure 7 is rotating in counterclockwise direction at an angular velocity of 5 rad/s. For the given instance, determine the angular velocity of link AB.
Solution

In ΔEBC,
Using Sine rule,
`(EB)/(sin(75)) - (BC)/(sin(45) )- (CE)/(sin(60)`
`EB = (200 xx sin (75))/(sin(45)) = 0.27 m CE = (200 xx sin (60))/sin(45) = 0.24m`
ωCD = VC x CD
`V_C = 5/0.1= 50` m/s
`ω_(BC) = V_C xx CE = 50 x 0.24 = 12` rad/s
`ω_(BC) = V_B xx EB`
`V_B = 12/(0.27) = 44.44`m/s
`ω_AB = V_B xx AB = 44.44 xx 0.15 = 6.666` rad/s
The angular velocity of link AB is 6.666 rad/s.
APPEARS IN
RELATED QUESTIONS
Rod AB of length 3 m is kept on a smooth plane as shown in the given figure.The velocity of end A is 5 m/s along the inclined plane.Locate the ICR and find velocity of end B.
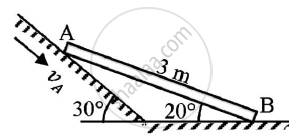
Given : Length of rod AB = 3m
va = 5 m/s
To find: ICR
Velocity of end B
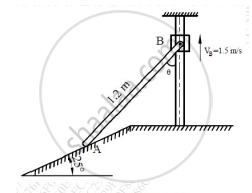
Using Instantaneous Centre of Rotation (ICR) method, find the velocity of point A for the instant shown in Figure 2. Collar B moves along the vertical rod, whereas link AB moves along the plane which is inclined at 250. Ɵ = 450