Advertisements
Advertisements
Question
The marks obtained by 100 students of a class in an examination are given below.
| Mark | No. of Student |
| 0 - 5 | 2 |
| 5 - 10 | 5 |
| 10 - 15 | 6 |
| 15 - 20 | 8 |
| 20 - 25 | 10 |
| 25 - 30 | 25 |
| 30 - 35 | 20 |
| 35 - 40 | 18 |
| 40 - 45 | 4 |
| 45 - 50 | 2 |
Draw 'a less than' type cumulative frequency curves (ogive). Hence find the median.
Solution
We first prepare the cumulative frequency table by less than method as given below–
| Marks | No. of students | Marks less than | Cumulative Frequency |
| 0 - 5 | 2 | 5 | 2 |
| 5 - 10 | 5 | 10 | 7 |
| 10 - 15 | 6 | 15 | 13 |
| 15 - 20 | 8 | 20 | 21 |
| 20 - 25 | 10 | 25 | 31 |
| 25 - 30 | 25 | 30 | 56 |
| 30 - 35 | 20 | 35 | 76 |
| 35 - 40 | 18 | 40 | 94 |
| 40 - 45 | 4 | 45 | 98 |
| 45 - 50 | 2 | 50 | 100 |
Thus we will plot the points (5, 2), (10, 7), (15, 13), (20, 21), (25, 31), (30, 56), (35, 76), (40, 94), (45, 98) and (50, 100).
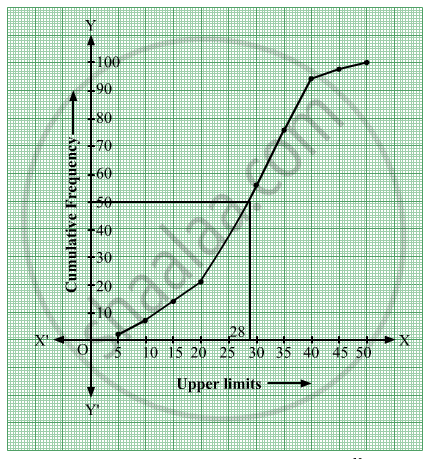
∴ From the above ogive, the horizontal line drawn from `("N")/(2)=50` intersects the ogive at a point whose x-coordinate is approximately 28.
∴ Hence, Median≈">≈28.
APPEARS IN
RELATED QUESTIONS
Find the median of the following data by making a ‘less than ogive’.
| Marks | 0 - 10 | 10-20 | 20 - 30 | 30 - 40 | 40 - 50 | 50 - 60 | 60 - 70 | 70 - 80 | 80-90 | 90-100 |
| Number of Students | 5 | 3 | 4 | 3 | 3 | 4 | 7 | 9 | 7 | 8 |
The table given below shows the weekly expenditures on food of some households in a locality
| Weekly expenditure (in Rs) | Number of house holds |
| 100 – 200 | 5 |
| 200- 300 | 6 |
| 300 – 400 | 11 |
| 400 – 500 | 13 |
| 500 – 600 | 5 |
| 600 – 700 | 4 |
| 700 – 800 | 3 |
| 800 – 900 | 2 |
Draw a ‘less than type ogive’ and a ‘more than type ogive’ for this distribution.
The following table gives the life-time (in days) of 100 electric bulbs of a certain brand.
| Life-tine (in days) | Less than 50 |
Less than 100 |
Less than 150 |
Less than 200 |
Less than 250 |
Less than 300 |
| Number of Bulbs | 7 | 21 | 52 | 9 | 91 | 100 |
Calculate the missing frequency form the following distribution, it being given that the median of the distribution is 24
| Age (in years) | 0 – 10 | 10 – 20 | 20 – 30 | 30 – 40 | 40 – 50 |
| Number of persons |
5 | 25 | ? | 18 | 7 |
The median of the distribution given below is 14.4 . Find the values of x and y , if the total frequency is 20.
| Class interval : | 0-6 | 6-12 | 12-18 | 18-24 | 24-30 |
| Frequency : | 4 | x | 5 | y | 1 |
The median of a given frequency distribution is found graphically with the help of
Calculate the mean of the following frequency distribution :
| Class: | 10-30 | 30-50 | 50-70 | 70-90 | 90-110 | 110-130 |
| Frequency: | 5 | 8 | 12 | 20 | 3 | 2 |
Look at the following table below.
| Class interval | Classmark |
| 0 - 5 | A |
| 5 - 10 | B |
| 10 - 15 | 12.5 |
| 15 - 20 | 17.5 |
The value of A and B respectively are?
Find the unknown entries a, b, c, d, e, f in the following distribution of heights of students in a class:
| Height (in cm) |
Frequency | Cumulative frequency |
| 150 – 155 | 12 | a |
| 155 – 160 | b | 25 |
| 160 – 165 | 10 | c |
| 165 – 170 | d | 43 |
| 170 – 175 | e | 48 |
| 175 – 180 | 2 | f |
| Total | 50 |
The following are the ages of 300 patients getting medical treatment in a hospital on a particular day:
| Age (in years) | 10 – 20 | 20 – 30 | 30 – 40 | 40 – 50 | 50 – 60 | 60 – 70 |
| Number of patients | 60 | 42 | 55 | 70 | 53 | 20 |
Form: Less than type cumulative frequency distribution.
