Advertisements
Advertisements
Question
The sides of a triangle are 21 cm, 17 cm, and 10 cm. Find its area.
Solution
Let a = 21 cm, b = 17 cm and c = 10 cm
∴ a + b + c
= 21 cm + 17 cm + 10 cm = 48 cm
s =`"a + b + c"/2=48/2` = 24 cm
Area of the triangle
= `sqrt(s(s-a)(s-b)(s-c))`
= `sqrt(24(24-21)(24-17)(24-10))`
= `sqrt(24xx3xx7xx14)`
= `sqrt(2xx2xx2xx3xx3xx7xx2xx7)`
= 2 × 2 × 3 × 7
= 84 cm2
APPEARS IN
RELATED QUESTIONS
A square lawn is surrounded by a path 2.5 m wide. If the area of the path is 165 m2 find the area of the lawn.
The base of a parallelogram is thrice it height. If its area is 768 cm2, find the base and the height of the parallelogram.
The adjacent sides of a parallelogram are 15 cm and 10 cm. If the distance between the longer sides is 6 cm, find the distance between the shorter sides.
The area of a rhombus is 84 cm2 and its perimeter is 56 cm. Find its height.
Find the base of a triangle whose area is 360 cm2 and height is 24 cm.
The legs of a right-angled triangle are in the ratio 4 : 3 and its area is 4056 cm2. Find the length of its legs.
The sides of a triangle are in the ratio 15 : 13 : 14 and its perimeter is 168 cm. Find the area of the triangle.
The diameter of a circle is 20 cm. Taking π = 3.14, find the circumference and its area.
The ratio between the radius of two circles is 5 : 7. Find the ratio between their:
(i) circumference
(ii) areas
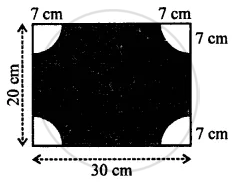
From each corner of a rectangular paper (30 cm x 20 cm) a quadrant of a circle of radius 7 cm is cut. Find the area of the remaining paper i.e., shaded portion.