Advertisements
Advertisements
Question
The zeroes of the quadratic polynomial x2 + kx + k, k ≠ 0 ______.
Options
Cannot both be positive
Cannot both be negative
Are always unequal
Are always equal
Solution
The zeroes of the quadratic polynomial x2 + kx + k, k ≠ 0 cannot both be positive.
Explanation:
Let p(x) = x2 + kx + k, k ≠ 0
On comparing p(x) with ax2 + bx + c, we get
Now, a = 1, b = k and c = k
`x = (-b +- sqrt(b^2 - 4ac))/(2a)` ....[By quadratic formula]
= `(-k +- sqrt(k^2 - 4k))/(2 xx 1)`
= `(-k +- sqrt(k(k - 4)))/2, k ≠ 0`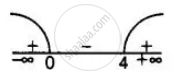
Here, we see that
k(k − 4) > 0
⇒ `k ∈ (-oo, 0) u (4, oo)`
Now, we know that
In quadratic polynomial ax2 + bx + c
If a > 0
b > 0
c > 0
or a < 0
b < 0
c < 0
Then the polynomial has always all negative zeroes.
And if a > 0, c < 0 or a < 0, c > 0
Then the polynomial has always zeroes of opposite sign
Case I: If `k ∈ (-oo, 0)`
i.e., k < 0
⇒ a = 1 > 0, b, c = k < 0
So, both zeroes are of opposite sign.
Case II: If `k ∈ (4, oo)`
i.e., k ≥ 4
⇒ a = 1 > 0, b, c > 4
So, both zeroes are negative.
Hence, in any case zeroes of the given quadratic polynomial cannot both be positive.
APPEARS IN
RELATED QUESTIONS
Find the zeroes of the polynomial `f(x) = x^2 ˗ 2x ˗ 8` and verify the relation between its zeroes and coefficients
Find the zeroes of the polynomial `x^2 – 3x – m(m + 3)`
If the zeroes of the polynomial `f(x) = x^3 – 3x^2 + x + 1` are (a – b), a and (a + b), find the values of a and b.
If one of the zeroes of the cubic polynomial x3 + ax2 + bx + c is -1, then the product of the
other two zeroes is ______.
If x3 + 11 is divided by x2 – 3, then the possible degree of remainder is ______.
If x4 + 3x2 + 7 is divided by 3x + 5, then the possible degrees of quotient and remainder are ______.
If one of the zeroes of the cubic polynomial x3 + px² + qx + r is -1, then the product of the other two zeroes is ______.
If the zeroes of the quadratic polynomial ax2 + bx + c, c ≠ 0 are equal, then ______.
Which of the following is not the graph of a quadratic polynomial?
The graph of y = f(x) is shown in the figure for some polynomial f(x).
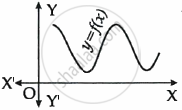
The number of zeroes of f(x) is ______.
