Advertisements
Advertisements
Question
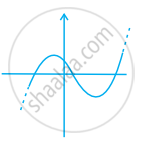
Which of the following is not the graph of a quadratic polynomial?
Options
Solution
Explanation:
For any quadratic polynomial ax2 + bx + c, a ≠ 0
The graph of the corresponding equation y = ax2 + bx + c has one of the two shapes either open upwards like ∪ or open downwards like ∩ depending on whether a > 0 or a < 0. These curves are called parabolas.
So, option (d) cannot be possible.
Also, the curve of a quadratic polynomial crosses the X-axis on at most two points but in option (d) the curve crosses the X-axis on the three points, so it does not represent the quadratic polynomial.
APPEARS IN
RELATED QUESTIONS
The graphs of y = p(x) are given in following figure, for some polynomials p(x). Find the number of zeroes of p(x).
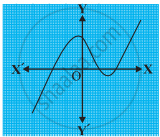
The graphs of y = p(x) are given in following figure, for some polynomials p(x). Find the number of zeroes of p(x).

Find the zeroes of the quadratic polynomial `f(x) = x^2 + 3x ˗ 10` and verify the relation between its zeroes and coefficients.
Find the zeroes of the quadratic polynomial `f(x) = 6x^2 – 3.`
If one of the zeroes of a quadratic polynomial of the form x² + ax + b is the negative of the other, then it ______.
If x3 + 1 is divided by x2 + 5, then the possible degree of quotient is ______.
If x3 + 11 is divided by x2 – 3, then the possible degree of remainder is ______.
A polynomial of degree n has ______.
Consider the following statements.
- x – 2 is a factor of x3 – 3x² + 4x – 4.
- x + 1 is a factor of 2x3 + 4x + 6.
- x – 1 is a factor of x5 + x4 – x3 + x² -x + 1.
In these statements
If the graph of a polynomial intersects the x-axis at exactly two points, it need not be a quadratic polynomial.