Advertisements
Advertisements
Question
Time taken by a construction company to construct a flyover is a normal variate with mean 400 labour days and a standard deviation of 100 labour days. If the company promises to construct the flyover in 450 days or less and agree to pay a penalty of ₹ 10,000 for each labour day spent in excess of 450. What is the probability that the company takes at most 500 days to complete the flyover?
Solution
Let x be a normal variate with mean 400 labour days and standard deviation of 100 labour days
m = 400 and σ = 100
The construction work should be completed within 450 days.
The standard normal variate
`(x - mu)/6 = (x - 400)/100`
Personality for 1 labour day = ₹ 10,000
P(at most 500 days) = P(X ≤ 500 )
When x = 500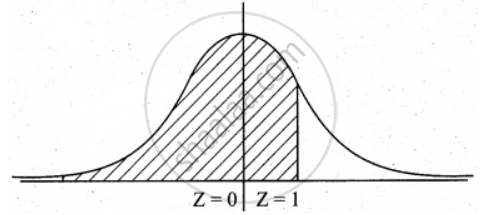
z = `(500 - 400)/100 = 100/100` = 1
P(X ≤ 500) = P(Z ≤ 1)
=P(`∞` < z < 0) – r – P(0 < z < 1)
= 0.5 + 0.3415
= 0.8413
APPEARS IN
RELATED QUESTIONS
Forty percent of business travellers carry a laptop. In a sample of 15 business travelers, what is the probability that 3 will have a laptop?
Forty percent of business travellers carry a laptop. In a sample of 15 business travelers, what is the probability that atleast three of the travelers have a laptop?
The mean of a binomial distribution is 5 and standard deviation is 2. Determine the distribution
Choose the correct alternative:
Which of the following cannot generate a Poisson distribution?
Choose the correct alternative:
The random variable X is normally distributed with a mean of 70 and a standard deviation of 10. What is the probability that X is between 72 and 84?
Choose the correct alternative:
In a large statistics class, the heights of the students are normally distributed with a mean of 172 cm and a variance of 25 cm. What proportion of students is between 165cm and 181 cm in height?
Choose the correct alternative:
The weights of newborn human babies are normally distributed with a mean of 3.2 kg and a standard deviation of 1.1 kg. What is the probability that a randomly selected newborn baby weight less than 2.0 kg?
Vehicles pass through a junction on a busy road at an average rate of 300 per hour. What is the expected number passing in two minutes?
The annual salaries of employees in a large company are approximately normally distributed with a mean of $50,000 and a standard deviation of $20,000. What percent of people earn more than $75,000
X is a normally distributed variable with mean µ = 30 and standard deviation σ = 4. Find P(X < 40)
