Advertisements
Advertisements
Question
Two adjacent angles of a parallelogram have equal measure. Find the measure of each of the angles of the parallelogram.
Solution

Let ABCD be a parallelogram, in which, let m∠A = m∠B = x
Since sum of two adjacent angles is 180°
∠A + ∠B = 180º
x + x = 180°
2x = 180°
x = 90º
∠C = ∠A = 90º (Opposite angles)
∠D = ∠B = 90º (Opposite angles)
Thus, each angle of the parallelogram measures 90º.
APPEARS IN
RELATED QUESTIONS
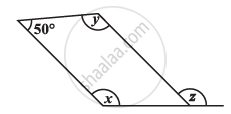
Consider the given parallelogram. Find the values of the unknowns x, y, z.
The following figure GUNS is a parallelogram. Find x and y. (Lengths are in cm)

If the ratio of measures of two adjacent angles of a parallelogram is 1 : 2, find the measures of all angles of the parallelogram.
Ratio of consecutive angles of a quadrilateral is 1 : 2 : 3 : 4. Find the measure of its each angle. Write, with reason, what type of a quadrilateral it is.
The given figure shows parallelogram ABCD. Points M and N lie in diagonal BD such that DM = BN.
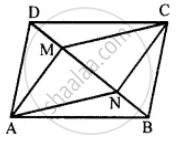
Prove that:
(i) ∆DMC = ∆BNA and so CM = AN
(ii) ∆AMD = ∆CNB and so AM CN
(iii) ANCM is a parallelogram.
The adjacent sides of a parallelogram are 5 cm and 9 cm. Its perimeter is ______.
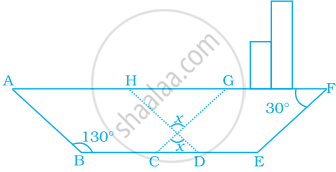
In the following figure of a ship, ABDH and CEFG are two parallelograms. Find the value of x.
Two angles of a quadrilateral are each of measure 75° and the other two angles are equal. What is the measure of these two angles? Name the possible figures so formed.
ABCD is a parallelogram. The bisector of angle A intersects CD at X and bisector of angle C intersects AB at Y. Is AXCY a parallelogram? Give reason.
A diagonal of a parallelogram bisects an angle. Will it also bisect the other angle? Give reason.
