Advertisements
Advertisements
Question
Two circles touch each other externally. The sum of their areas is 58π cm2 and the distance between their centers is 10 cm. Find the radii of the two circles.
Solution
Let r and R be the radius of the two circles.
r + R = 10 ....(1)
πr2 + πR2 = 58π .....(2)
Putting the value of r in (2)
r2 + R2 = 58
( 10 - R )2 + R2 = 58
100 - 20R + R2 + R2 = 58
2R2 - 20R + 42 = 0
R2 - 10R + 21 = 0
( R - 3 )( R - 7 ) = 0
R = 3, 7.
Hence the radius of the two circles is 3cm and 7cm respectively.
APPEARS IN
RELATED QUESTIONS
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the length of the arc. (Use π = `22/7`)
Find the area of the circle in which a square of area 64 cm2 is inscribed. [Use π = 3.14]
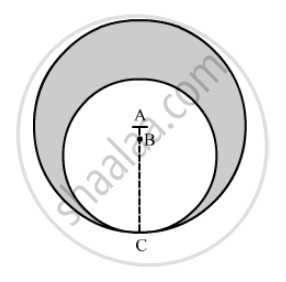
In the following figure, two circles with centres A and B touch each other at the point C. If AC = 8 cm and AB = 3 cm, find the area of the shaded region.
The circumference of a circle is 100 m. The side of a square inscribed in the circle is
If the perimeter of a semi-circular protractor is 36 cm, then its diameter is
The perimeter of the sector OAB shown in the following figure, is

If the area of a sector of a circle bounded by an arc of length 5π cm is equal to 20π cm2, then the radius of the circle
Find the lengths of the arcs cut off from a circle of radius 12 cm by a chord 12 cm long. Also, find the area of the minor segment.
Find the area enclosed between two concentric circles, If their radii are 6cm and 13cm respectively.
A pendulum swings through an angle of 30∘ and describes an arc 8.8 cm in length. Find the length of the pendulum in cm.
