Advertisements
Advertisements
Question
The perimeter of the sector OAB shown in the following figure, is

Options
\[\frac{64}{3} cm\]
26 cm
\[\frac{64}{5} cm\]
19 cm
Solution
We know that perimeter of a sector of radius `r=2r+θ/360xx2pir`
We have given sector angle and radius of the sector and we are asked to find perimeter of the sector OAB.
Therefore, substituting the corresponding values of the sector angle and radius in equation (1) we get,
Perimeter=`2xx7+60/360xx2pixx7c`
We will simplify equation (2) as shown below,
Perimeter=`2xx7+1/6xx2pixx7`
Substituting `pi=22/7` we get
Perimeter=`2xx7+1/6xx2xx22/7xx7`
∴ Perimeter =`2xx7+1/6xx2xx22`
∴ Perimeter =`2xx7+1/3xx22`
∴ Perimeter=`2xx7+22/3`
∴ Perimeter=`14+22/3`
Now we will make the denominator same.
∴ Perimeter=`42/3+22/3`
∴ Perimeter =` (42+22)/3`
∴ Perimeter=`64/3`
Therefore, perimeter of the sector is `64/3 cm`
APPEARS IN
RELATED QUESTIONS
A circular flower garden has an area of 314 m2. A sprinkler at the centre of the garden can cover an area that has a radius of 12 m. Will the sprinkler water the entire garden? (Take π = 3.14)
The area of a parallelogram is `392m^2` . If its altitude is twice the corresponding base, determined the base and the altitude.
What is the area of a sector of a circle of radius 5 cm formed by an arc of length 3.5 cm?
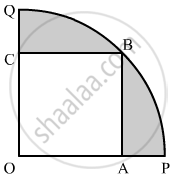
In the given figure, a square OABC is inscribed in a quadrant OPBQ of a circle. If OA = 20 cm, find the area of the shaded region. [Use π = 3.14.]
Find the area of the shaded region in the given figure, where a circular arc of radius 6 cm has been drawn with vertex of an equilateral triangle of side 12 cm as centre and a sector of circle of radius 6 cm with centre B is made.
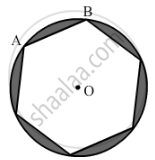
A round table cover has six equal designs as shown in the given figure. If the radius of the cover is 35 cm, then find the total area of the design.
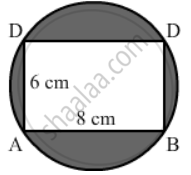
In the given figure, ABCD is a rectangle inscribed in a circle having length 8 cm and breadth 6 cm. If π = 3.14, then the area of the shaded region is:
The diameter of a wheel is 0.70 m. Find the distance covered by it in 500 revolutions. If the wheel takes 5 minutes to make 500 revolutions; find its speed in :
(i) m/s
(ii) km/hr.
Find the area of the circle, length of whose circumference is equal to the sum of the lengths of the circumferences with radii 15 cm and 13 cm.
The ratio of the area of a circle to the area of its semicircle is
