Advertisements
Advertisements
Question
Two cylinders A and B are formed by folding a rectangular sheet of dimensions 20 cm × 10 cm along its length and also along its breadth respectively. Then volume of A is ______ of volume of B.
Solution
Two cylinders A and B are formed by folding a rectangular sheet of dimensions 20 cm × 10 cm along its length and also along its breadth respectively. Then volume of A is twice of volume of B
Explanation:
We have, a rectangular sheet of dimensions 20 cm × 10 cm.
If we fold it along its length, which is 20 cm, then the resultant figure is a cylinder with height, h = 10 cm and base circumference, 2πr = 20 cm
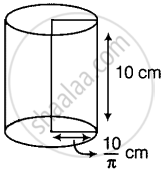
⇒ `r = 20/(2pi) = 10/pi cm`
∴ The volume of the cylinder, so formed = πr2h
= `pi xx 10/pi xx 10/pi xx 10`
= `1000/pi cm^3`
= V1 (say) ...(i)
Again, if we fold the rectangular sheet along its breadth, which is 10 cm, the figure so obtained is a cylinder with height h = 20 cm and the base circumference 2πr = 10 cm
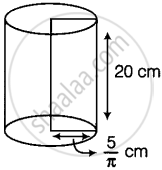
⇒ `r = 10/(2pi) = 5/pi cm`
∴ Volume of the cylinder = πr2h
= `pi xx 5/pi xx 5/pi xx 20`
= `500/pi cm^3`
= V2 (say) ...(ii)
i.e. V2 = 2V1
From equations, (i) and (ii), we see that the volume of A is twice the volume of B.
APPEARS IN
RELATED QUESTIONS
A vessel is a hollow cylinder fitted with a hemispherical bottom of the same base. The depth of the cylindrical part is `4 2/3` m and the diameter of hemisphere is 3.5 m. Calculate the capacity and the internal surface area of the vessel.
An open cylindrical vessel of internal diameter 7 cm and height 8 cm stands on a horizontal table. Inside this is placed a solid metallic right circular cone, the diameter of whose base is `3 1/2` cm and height 8 cm. Find the volume of water required to fill the vessel. If this cone is replaced by another cone, whose height is `1 3/4` cm and the radius of whose base is 2 cm, find the drop in the water level.
An exhibition tent is in the form of a cylinder surmounted by a cone. The height of the tent above the ground is 85 m and height of the cylindrical part of 50 m. If the diameter of the base is 168 m, find the quantity of canvas required to make the tent. Allow 20% extra for fold and for stitching. Give your answer to the nearest m2.
How much iron is needed to make a rod of length 90 cm and diameter 1.4 cm?
Find the volume of the largest cylinder formed when a rectangular piece of paper 44 cm by 33 cm is rolled along it : longer side.
Water flows through a cylindrical pipe of internal diameter 7 cm at 36 km/hr. Calculate the time in minutes it would take to fill the cylindrical tank, the radius of whose base is 35 cm, and height is 1 m.
In a hollow cylinder, the sum of the external and internal radii is 14 cm and the width is 4 cm. If its height is 20 cm, the volume of the material in it is
The water for a factory is stored in a hemispherical tank whose internal diameter is 14 m. The tank contains 50 kilolitres of water. Water is pumped into the tank to fill to its capacity. Calculate the volume of water pumped into the tank.
Radius of a cylinder is r and the height is h. Find the change in the volume if the height remains same and the radius is halved.
Volume of a cylinder of height 3 cm is a 48π. Radius of the cylinder is ______.
