Advertisements
Advertisements
Question
Two sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of ΔPQR (see the given figure). Show that:
- ΔABM ≅ ΔPQN
- ΔABC ≅ ΔPQR
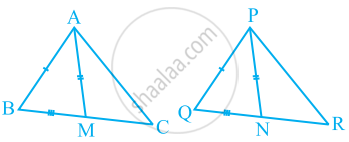
Solution
In ΔABC, AM is the median to BC.
∴ BM = `1/2 BC`
In ΔPQR, PN is the median to QR.
∴ QN = `1/2QR`
However, BC = QR
∴ `1/2BC` = `1/2QR`
⇒ BM = QN …(1)
(i) In ΔABM and ΔPQN,
AB = PQ ...(Given)
BM = QN ...[From equation (1)]
AM = PN ...(Given)
∴ ΔABM ≅ ΔPQN ...(SSS congruence rule)
∠ABM = ∠PQN ...(By corresponding parts of congruent triangles)
∠ABC = ∠PQR …(2)
(ii) In ΔABC and ΔPQR,
AB = PQ ...(Given)
∠ABC = ∠PQR ...[From equation (2)]
BC = QR ...(Given)
⇒ ΔABC ≅ ΔPQR ...(By SAS congruence rule)
APPEARS IN
RELATED QUESTIONS
ABC is a right angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
AD is an altitude of an isosceles triangles ABC in which AB = AC. Show that
- AD bisects BC
- AD bisects ∠A
Prove that in a quadrilateral the sum of all the sides is greater than the sum of its diagonals.
In the following figure, BA ⊥ AC, DE ⊥ DF such that BA = DE and BF = EC. Show that ∆ABC ≅ ∆DEF.

ABC is an isosceles triangle in which AC = BC. AD and BE are respectively two altitudes to sides BC and AC. Prove that AE = BD.
Prove that sum of any two sides of a triangle is greater than twice the median with respect to the third side.
In a right triangle, prove that the line-segment joining the mid-point of the hypotenuse to the opposite vertex is half the hypotenuse.
Line segment joining the mid-points M and N of parallel sides AB and DC, respectively of a trapezium ABCD is perpendicular to both the sides AB and DC. Prove that AD = BC.
ABCD is a quadrilateral such that diagonal AC bisects the angles A and C. Prove that AB = AD and CB = CD.
ABC is a right triangle such that AB = AC and bisector of angle C intersects the side AB at D. Prove that AC + AD = BC.
