Advertisements
Advertisements
Question
Use the mirror equation to deduce that the virtual image produced by a convex mirror is always diminished in size and is located between the focus and the pole.
Solution
For a convex mirror, the focal length (f) is positive.
∴ f > 0
When the object is placed on the left side of the mirror, the object distance (u) is negative,
∴ u < 0
For image distance v, we have the mirror formula:
`1/"v" + 1/"u" = 1/"f"`
`1/"v" = 1/"f" - 1/"u"`
But we have u < 0
∴ `1/"v" > 1/"f"`
v < f
Hence, the image formed is diminished and is located between the focus (f) and the pole.
APPEARS IN
RELATED QUESTIONS
a) Give two reasons to explain why reflecting telescopes are preferred over refracting type.
Use the mirror equation to show that an object placed between f and 2f of a concave mirror produces a real image beyond 2f.
Use the mirror equation to deduce that an object placed between the pole and focus of a concave mirror produces a virtual and enlarged image.
Using mirror formula, explain why does a convex mirror always produce a virtual image.
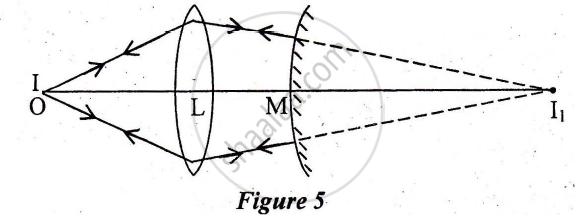
A point object O is placed at a distance of 15cm from a convex lens L of focal length 1 Ocm as shown in Figure 5 below. On the other side of the lens, a convex mirror M is placed such that its distance from the lens is equal to the focal length of the lens. The final image formed by this combination is observed to coincide with the object O. Find the focal length of the convex mirror
Use the mirror equation to show that an object placed between f and 2f of a concave mirror forms an image beyond 2f.
Define the term 'limit of resolution'?
A cylindrical vessel of diameter 12 cm contains 800π cm3 of water. A cylindrical glass piece of diameter 8.0 cm and height 8.0 cm is placed in the vessel. If the bottom of the vessel under the glass piece is seen by the paraxial rays (see figure), locate its image. The index of refraction of glass is 1.50 and that of water is 1.33.

A small object is placed at the centre of the bottom of a cylindrical vessel of radius 3 cm and height 4 cm filled completely with water. Consider the ray leaving the vessel through a corner. Suppose this ray and the ray along the axis of the vessel are used to trace the image. Find the apparent depth of the image and the ratio of real depth to the apparent depth under the assumptions taken. Refractive index of water = 1.33.
Find the maximum angle of refraction when a light ray is refracted from glass (μ = 1.50) to air.
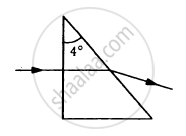
Find the angle of deviation suffered by the light ray shown in figure. The refractive index μ = 1.5 for the prism material.
Name the physical principle on which the working of optical fibers is based.
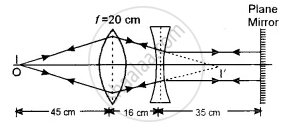
The figure below shows the positions of a point object O, two lenses, a plane mirror and the final image I which coincides with the object. The focal length of the convex lens is 20 cm. Calculate the focal length of the concave lens.
The focal length f is related to the radius of curvature r of the spherical convex mirror by ______.
Car B overtakes car A at a relative speed of 40 ms-1. How fast will the image of car B appear to move in the mirror of focal length 10 cm fitted in car A, when car B is 1.9 m away from car A?
A point object is placed at a distance of 30 cm from a convex mirror of a focal length of 30 cm. What is the separation between the image and the object?
A convex lens of focal length 15 cm is placed coaxially in front of a convex mirror. The lens is 5 cm from the pole of the mirror. When an object is placed on the axis at a distance of 20 cm from the lens, it is found that the image coincides with the object. Calculate the radius of curvature of the mirror - (consider all-optical event):
