Advertisements
Advertisements
Question
Using mirror formula, explain why does a convex mirror always produce a virtual image.
Solution
For a convex mirror,
Focal length, f > 0
Position of object, u < 0
From mirror formula, we have
`1/f=1/v+1/u=>1/v=1/f-1/u`
`=>1/v>0`
∴v>0
This means that the image formed by a convex lens is always behind the mirror and hence, virtual.
RELATED QUESTIONS
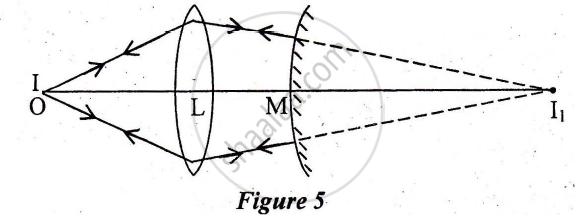
A point object O is placed at a distance of 15cm from a convex lens L of focal length 1 Ocm as shown in Figure 5 below. On the other side of the lens, a convex mirror M is placed such that its distance from the lens is equal to the focal length of the lens. The final image formed by this combination is observed to coincide with the object O. Find the focal length of the convex mirror
Define the term 'limit of resolution'?
Use the mirror equation to show a convex mirror always produces a virtual image independent of the location of the object ?
The rays of different colours fail to converge at a point after going through a converging lens. This defect is called
A light ray falling at an angle of 45° with the surface of a clean slab of ice of thickness 1.00 m is refracted into it at an angle of 30°. Calculate the time taken by the light rays to cross the slab. Speed of light in vacuum = 3 × 108 m s−1.
For paraxial rays, show that the focal length of a spherical mirror is one-half of its radius of curvature.
A parallel beam of light is allowed to fall on a transparent spherical globe of diameter 30cm and refractive index 1.5. The distance from the centre of the globe at which the beam of light can converge is ______ mm.
The focal length f is related to the radius of curvature r of the spherical convex mirror by ______.
Car B overtakes car A at a relative speed of 40 ms-1. How fast will the image of car B appear to move in the mirror of focal length 10 cm fitted in car A, when car B is 1.9 m away from car A?
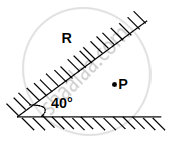
Two plane mirrors are inclined at an angle of 40°. The possible number of images of an object placed at point P would be?