Advertisements
Advertisements
Question
Using the measures of the angles given in the figure alongside, find the measures of the remaining three angles.

Solution
In the given figure,
∠BOC = ∠FOE = 4y (Vertically opposite angles)
∠EOD = ∠AOB = 8y (Vertically opposite angles)
∠AOF = ∠COD = 6y (Vertically opposite angles)
Now, ∠AOB + ∠BOC + ∠COD = 180∘ (Linear Pair angles)
⇒ 8y + 4y + 6y = 180∘
⇒ 18y = 180∘
⇒ y = 10∘
Therefore,
∠BOC = 4y
= 4 × 10∘
= 40∘
∠EOD = 8y
= 8 × 10∘
= 80∘
∠AOF = 6y
= 6 × 10∘
= 60∘
Hence, the measures of ∠BOC, ∠EOD, ∠AOF are 40∘, 80∘ and 60∘ respectively.
RELATED QUESTIONS
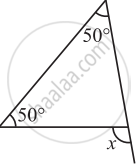
Find the value of the unknown exterior angle x in the following diagram:
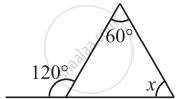
Find the value of the unknown interior angle x in the following figure.
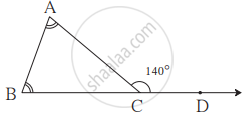
∠ACD is an exterior angle of ∆ABC. The measures of ∠A and ∠B are equal. If m∠ACD = 140°, find the measures of the angles ∠A and ∠B.
Using the diagram find the value of x.
If an exterior angle of a triangle is 115° and one of the interior opposite angles is 35°, then the other two angles of the triangle are
In ∆PQR, if ∠P = 60°, and ∠Q = 40°, then the exterior angle formed by producing QR is equal to ______.
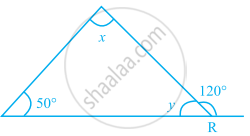
The measures of ∠x and ∠y in the given figure are respectively.
The sum of an exterior angle of a triangle and its adjacent angle is always ______.
In the given figure QP || RT. Find the values of x and y.
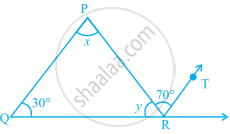
Find the value of x in the given figure.

