Advertisements
Advertisements
Question
In the following figure, an equilateral triangle ABC of side 6 cm has been inscribed in a circle. Find the area of the shaded region. (Take π = 3.14).
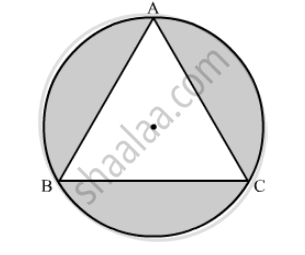
Solution
We have to find the area of the shaded portion. We have`ΔABC`which is an equilateral triangle and`AB=6 cm`. Let r be the radius of the circle.
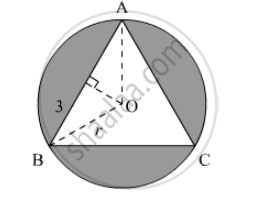
We have O as the circumcentre.
`∠OBA=30°`
So,
`Cos (30°)=3/r`
Thus, r=`2sqrt3`
So area of the shaded region,
=Area of the circle-ar(ΔABC)
`=(3.14)(2sqrt3)^2-sqrt3/4(6)^2`
`=(37.68-15.59)cm^2`
`= 22.126cm^2`
APPEARS IN
RELATED QUESTIONS
The length and breadth of a rectangular garden are in the ratio 9:5. A path 3.5 m wide, running all around inside it has an area of `1911m^2` . Find the dimensions of the garden.
The adjacent sides of a parallelogram are 32 cm and 24 cm. If the distance between the longer sides is 17.4 cm, find the distance between the shorter sides.
Prove that the area of a circular path of uniform width h surrounding a circular region of radius r is `pih(2r+h)`
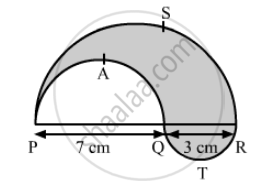
In the the following figure, PSR, RTQ and PAQ are three semicircles of diameter 10 cm, 3 cm and 7 cm respectively. Find the perimeter of shaded region.
In the following figure, the boundary of the shaded region consists of four semi-circular arcs, the smallest two being equal. If the diameter of the largest is 14 cm and of the smallest is 3.5 cm, find
the length of the boundary.
the area of the shaded region.

If the area of a sector of a circle bounded by an arc of length 5π cm is equal to 20π cm2, then its radius is
If a chord of a circle of radius 28 cm makes an angle of 90 ° at the centre, then the area of the major segment is
The area of a circle is 98.56 cm2. Find its circumference.
Diameter of a circular garden is 9.8 m. Find its area.
If the perimeter of a semicircular protractor is 72 cm where `pi = 22/7`, then the diameter of protractor is ____________.
