Advertisements
Advertisements
Question
A circle is inscribed in an equilateral triangle ABC is side 12 cm, touching its sides (the following figure). Find the radius of the inscribed circle and the area of the shaded part.

Solution
We have to find the area of the shaded portion. We have`ΔABC`which is an equilateral triangle and`AB=12 cm`.

We have O as the incentre and OP, OQ and OR are equal.
So,
`ar(Δ ABC)=ar(ΔOAB)+ar(ΔOBC)+ae(ΔOCA)`
Thus,
`sqrt3/4(12)^2=3(1/2(12))(r)`
`r=(36sqrt3)/18 cm`
`=2sqrt3 cm`
So area of the shaded region,
`= ar (Δ ABC)-Area of the circle`
`=sqrt3/4(12)^2-22/7(2sqrt3)^2`
`=(62.35-37.71)cm^2`
`=24.64 cm^2`
APPEARS IN
RELATED QUESTIONS
From a circular card sheet of radius 14 cm, two circles of radius 3.5 cm and a rectangle of length 3 cm and breadth 1 cm are removed. (as shown in the following figure). Find the area of the remaining sheet. (Take `pi = 22/7`)

Find the length of the hypotenuse of an isosceles right-angled triangle whose area is `200^2` cm . Also, find its perimeter
A room is 16 m long and 13.5 m broad. Find the cost of covering its floor with 75-m-wide carpet at ₹ 60 per metre.
The area of a square filed is 8 hectares. How long would a man take to cross it diagonally by walking at the rate of 4 km per hour?
A circular park has a path of uniform width around it. The difference between the outer and inner circumferences of the circular path is 132 m. Its width is
The radius of a wheel is 0.25 m. The number of revolutions it will make to travel a distance of 11 km will be
The minute hand of a clock is 15 cm long. Calculate the area swept by it in 20 minutes.
The area of a circle is 98.56 cm2. Find its circumference.
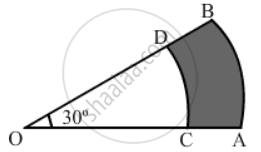
In the given figure, the sectors of two concentric circles of radii 7 cm and 3.5 cm are shown. Find the area of the shaded region.
A lawn is in the shape of a semicircle of diameter 42m. the lawn is surrounded by a flower bed of width 7m all round. Find the area of the flower bed in m2.
