Advertisements
Advertisements
Question
A circular park has a path of uniform width around it. The difference between the outer and inner circumferences of the circular path is 132 m. Its width is
Options
20 m
21 m
22 m
24 m
Solution

Let OA = r be the radius of the inner circle and OB = r′ be the radius of the outer circle.
Therefore, circumference of the inner circle =`2pir` and circumference of the outer circle`=2pir'`
Here we have to find the width of the circular park that is we have to find `r'-r.`.
We have given the difference between the circumferences of outer circle and inner circle.
`∴ 2pir'-2pir=132`
`∴2pi(r'-r)=132`
Substituting `pi=22/7` we get,
`2xx22/7xx(r'-r)=132`
Now we will multiply both sides of the equation by `7/44`
`(r'-r)=132xx7/44`
`∴(r'-r)=3xx7`
`∴(r'-r)=21`
Therefore, the width is`21 m`.
APPEARS IN
RELATED QUESTIONS
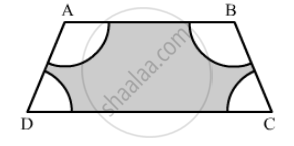
In the given figure, ABCD is a trapezium with AB || DC, AB = 18 cm DC = 32 cm and the distance between AB and DC is 14 cm. Circles of equal radii 7 cm with centres A, B, C and D have been drawn. Then find the area of the shaded region.
(Use \[\pi = \frac{22}{7}\]
If the circumference and the area of a circle are numerically equal, then diameter of the circle is
The ratio of the outer and inner perimeters of a circular path is 23 : 22. If the path is 5 metres wide, the diameter of the inner circle is
The area of the largest triangle that can be inscribed in a semi-circle of radius r is
If area of a circle inscribed in an equilateral triangle is 48π square units, then perimeter of the triangle is
If the area of a circle is equal to the sum of the areas of two circles of diameters 10 cm and 24 cm, then diameter of the large circle (in cm) is
The perimeter of a certain sector of a circle of radius 6.5 cm in 31 cm. Find the area of the sector.
On increasing the diameter of a circle by 40%, its area will be increased by
Find the area of a flat circular ring formed by two concentric circles (circles with the same centre) whose radii are 9 cm and 5 cm.
If the perimeter of a semicircular protractor is 72 cm where `pi = 22/7`, then the diameter of protractor is ____________.
