Advertisements
Advertisements
Question
If the circumference and the area of a circle are numerically equal, then diameter of the circle is
Options
\[\frac{\pi}{2}\]
2\[\frac{\pi}{2}\]
2
4
Solution
We have given that circumference and area of a circle are numerically equal.
Let it be x.
Let r be the radius of the circle, therefore, circumference of the circle is `2pir`and area of the circle will be `pir^2`.
Therefore, from the given condition we have,
`2pir=x .................(1)`
`pi r^2 = x ...............(2)`
Therefore, from equation (1) get `r=x/(2pi)`. Now we will substitute this value in equation
we get, `pi(x/(2pi))^2=x`
Simplifying further we get,
`pixx x^2/(4pi^2)=x`
Cancelling x we get,
`pi xx x/(4pi^2)=1`
Now we will cancel `pi`
`x/(4pi)=1...........(3)`
Now we will multiply both sides of the equation (3) by `4pi` we get, `x=4pi`
We can rewrite this equation as given below, `x=2xxpixx2`
Comparing equation (4) with equation (1) we get r = 2.
Therefore, radius of the circle is 2. We know that diameter of the circle is twice the radius of the circle.
`∴ "diameter"=2xx "radius"`
`∴ "diameter"=2xx2`
`∴"diameter"=4`
Therefore, diameter of the circle is 4.
Hence, option (d) is correct.
APPEARS IN
RELATED QUESTIONS
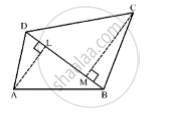
In the given figure ABCD is quadrilateral in which diagonal BD = 24 cm, AL ⊥ BD and CM ⊥ BD such that AL = 9cm and CM = 12 cm. Calculate the area of the quadrilateral.
An archery target has three regions formed by three concentric circles as shown in figure. If the diameters of the concentric circles are in the ratios 1 : 2 : 3, then find the ratio of the areas of three regions.
From a circular piece of cardboard of radius 3 cm two sectors of 900 have been cutoff . Find the perimeter of the remaining portion nearest hundredth centimeters ( `"Take" pi = 22/ 7`).
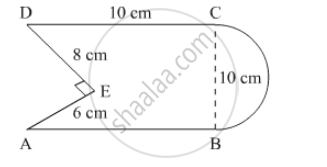
Find the area of the following figure, in square cm, correct to one place of decimal. (Take π = 22/7).
Find the area of a shaded region in the the following figure,where a circular arc of radius 7 cm has been drawn with vertex A of an equilateral triangle ABC of side 14 cm as centre. (Use π = 22/7 and \[\sqrt{3}\] = 1.73)

In the following figure, shows the cross-section of railway tunnel. The radius OA of the circular part is 2 m. If ∠AOB = 90°, calculate:
the height of the tunnel
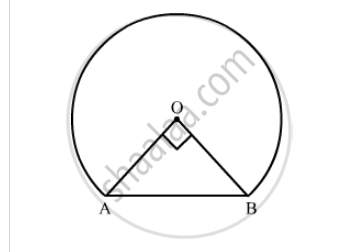
If a square is inscribed in a circle, what is the ratio of the areas of the circle and the square?
The areas of two concentric circles are 1386 cm2 and 962.5 cm2. The width of the ring is
The following figure shows two circles with the same center. The radius of the larger circle is 10 cm and the radius of the smaller circle is 4 cm.

Find:
(a) the area of the larger circle
(b) the area of the smaller circle
(c) the shaded area between the two circles. (π = 3.14)
Area of the circle obtained in 196 m2 is ______.
