Advertisements
Advertisements
Question
The radius of a wheel is 0.25 m. The number of revolutions it will make to travel a distance of 11 km will be
Options
2800
4000
5500
7000
Solution
We have given the radius of the wheel that is 0.25 cm.
We know that distance covered by the wheel in one revolution=`" Distance moved"/"Number of revolutions"`
Distance covered by the wheel in one revolution is equal to the circumference of the wheel.
`2pi r="Distance moved"/"Number of revolutions"......(1)`
Distance moved is given as 11 km so we will first convert it to m.
∴ 11 km = 11000 m
Now we will substitute the values in equation (1),
`2xxpixx0.25=11000/"Number of revalution"`
Now we will substitute pi=`22/7`
`2xx22/7xx0.25=11000/"Number of revolutions"`
Simplifying equation (1) we get,
`∴" Number of revolutions"=(11000xx7)/(2xx22xx0.25)`
`∴" Number of revolutions"=(11000xx7)/(22xx0.5)`
`∴" Number of revolutions"=(1000xx7)/(2xx0.5)`
`∴" Number of revolutions"=7000/1`
`∴" Number of revolutions"=7000`
Therefore, it will make `7000` revolutions to travel a distance of 11 km.
APPEARS IN
RELATED QUESTIONS
Find the length of the hypotenuse of an isosceles right-angled triangle whose area is `200^2` cm . Also, find its perimeter
The area of a square filed is 8 hectares. How long would a man take to cross it diagonally by walking at the rate of 4 km per hour?
The outer circumference of a circular race-track is 528 m . The track is everywhere 14 m wide. Calculate the cost of levelling the track at the rate of 50 paise per square metre.
`(use pi=22/7). `
In the following figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the (i) quadrant OACB (ii) shaded region.

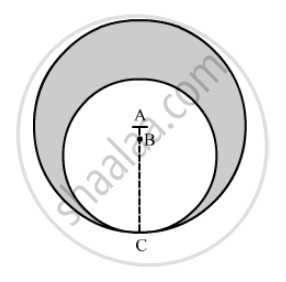
In the following figure, two circles with centres A and B touch each other at the point C. If AC = 8 cm and AB = 3 cm, find the area of the shaded region.
Area of the largest triangle that can be inscribed in a semi-circle of radius r units is ______.
The minute hand of a clock is 12 cm long. Find the area swept by in it 35 minutes.
The minute hand of a clock is 8 cm long. Find the area swept by the minute hand between 8.30 a.m. and 9.05 a.m.
A steel wire, when bent in the form of a square, encloses an area of 121 cm2. The same wire is bent in the form of a circle. Find area the circle.
The circumference of a circular table is 88 m. Find its area.
