Advertisements
Advertisements
Question
What does a toroid consist of? Find out the expression for the magnetic field inside a toroid for N turns of the coil having the average radius r and carrying a current I. Show that the magnetic field in the open space inside and exterior to the toroid is zero.
Solution
Toroid is a hollow circular ring on which a large number of turns of a wire are closely wound.
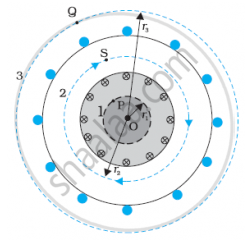
Figure shows a sectional view of the toroid. The direction of the magnetic field inside is clockwise as per the right-hand thumb rule for circular loops. Three circular Amperian loops 1, 2 and 3 are shown by dashed lines.
By symmetry, the magnetic field should be tangential to each of them and constant in magnitude for a given loop.
Let the magnetic field inside the toroid be B. We shall now consider the magnetic field at S. Once again we employ
Ampere’s law in the form of,
`ointvecB.dvecI = μ_0I` Or, BL = μ0NI
Where, L is the length of the loop for which B is tangential, I be the current enclosed by the loop and N be the number of turns.
We find, L = 2πr.
The current enclosed I is (for N turns of toroidal coil) N I.
B (2πr) = µ0NI , therefore, `B = (μ_0NI)/(2pir)`
Open space inside the toroid encloses no current thus, I = 0.
Hence, B = 0
Open space exterior to the toroid:
Each turn of current carrying wire is cut twice by the loop 3. Thus, the current coming out of the plane of the paper is cancelled exactly by the current going into it. Thus,
I= 0, and B = 0.
APPEARS IN
RELATED QUESTIONS
Express Biot – Savart law in the vector form.
State Biot-Savart law.
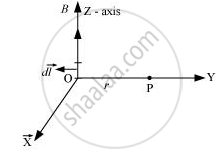
A current I flows in a conductor placed perpendicular to the plane of the paper. Indicate the direction of the magnetic field due to a small element d `vecl` at point P situated at distance `vecr` from the element as shown in the figure.
A wire of length l is bent in the form of an equilateral triangle and carries an electric current i. Find the magnetic field B at the centre.
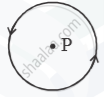
An electric current I flows through a circular loop as shown in Figure 2(b) below. Write an expression and direction for the magnetic field at the centre of the loop at point P.
Derive the expression for the magnetic field due to a current carrying coil of radius r at a distance x from the centre along the X-axis.
A straight wire carrying a current of 5 A is bent into a semicircular arc of radius 2 cm as shown in the figure. Find the magnitude and direction of the magnetic field at the centre of the arc.

A straight wire carrying a current of 5 A is bent into a semicircular arc radius 2 cm as shown in the figure. Find the magnitude and direction of the magnetic field at the center of the arc

Two concentric circular loops of radius 1 cm and 20 cm are placed coaxially.
(i) Find mutual inductance of the arrangement.
(ii) If the current passed through the outer loop is changed at a rate of 5 A/ms, find the emf induced in the inner loop. Assume the magnetic field on the inner loop to be uniform.
- both are long range and inversely proportional to the square of distance from the source to the point of interest.
-
both are linear in source.
-
both are produced by scalar sources.
-
both follow principle of superposition.
Biot-Savart law indicates that the moving electrons velocity (V) produce a magnetic field B such that ______.
