Advertisements
Advertisements
प्रश्न
1.0 mol of a monoatomic ideal gas is expanded from state (1) to state (2) as shown in figure. Calculate the work done for the expansion of gas from state (1) to state (2) at 298 K.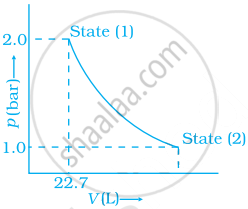
उत्तर
It is clear from the figure that the process has been carried out in infinite steps, hence it is isothermal reversible expansion.
w = – 2.303 nRT log `V_2/V_1`
But, p1V1 = p2V2
⇒ `V_2/V_1 = p_1/p_2 = 2/1` = 2
∴ w = – 2.303 nRT log `p_1/p_2`
= – 2.303 × 1 mol × 8.314 J mol–1 K–1 × 298 K–1 × log 2
= – 2.303 × 8.314 × 298 × 0.3010 J
= –1717.46 J
APPEARS IN
संबंधित प्रश्न
The pressure-volume work for an ideal gas can be calculated by using the expression w = `- int_(v_i)^(v_f) p_(ex) dV`. The work can also be calculated from the pV– plot by using the area under the curve within the specified limits. When an ideal gas is compressed (a) reversibly or (b) irreversibly from volume Vi to Vf. choose the correct option.
For an ideal gas, the work of reversible expansion under isothermal condition can be calculated by using the expression w = `- nRT` In `V_f/V_i`. A sample containing 1.0 mol of an ideal gas is expanded isothermally and reversibly to ten times of its original volume, in two separate experiments. The expansion is carried out at 300 K and at 600 K respectively.
(i) Work done at 600 K is 20 times the work done at 300 K.
(ii) Work done at 300 K is twice the work done at 600 K.
(iii) Work done at 600 K is twice the work done at 300 K.
(iv) ∆U = 0 in both cases.
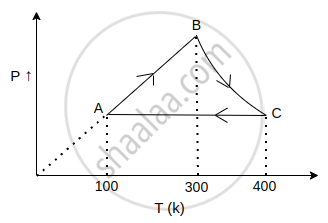
A sample of 1.0 mol of a monoatomic ideal gas is taken through a cyclic process of expansion and compression as shown in figure 6.1. What will be the value of ∆H for the cycle as a whole?

Represent the potential energy/enthalpy change in the following processes graphically.
(a) Throwing a stone from the ground to roof.
(b) \[\ce{1/2 H2(g) + 1/2 Cl2 (g) ⇌ HCl (g) Δ_rH^Θ = - 92.32 kJ mol^{-1}}\]
In which of the processes potential energy/enthalpy change is contributing factor to the spontaneity?
An ideal gas is allowed to expand against a constant pressure of 2 bar from 10 L to 50 L in one step. Calculate the amount of work done by the gas. If the same expansion were carried out reversibly, will the work done be higher or lower than the earlier case? (Given that 1 L bar = 100 J)
Match the following :
| Column I | Column II |
| (i) Entropy of vapourisation | (a) decreases |
| (ii) K for spontaneous process | (b) is always positive |
| (iii) Crystalline solid state | (c) lowest entropy |
| (iv) ∆U in adiabatic expansion of ideal gas | (d) `(∆H_(vap))/T_b` |
The net work done in the following cycle for one mol of an ideal gas will be ______ (in calorie), where in process BC, PT = constant. (R = 2 cal/mol-K).
1 mole of an ideal monoatomic gas initially at 1 atm and 300 K experiences a process by which pressure is doubled. The nature of the process is unspecified but 6. ΔU = 900 cal. The final volume will be ______ l.
[Given : R = 0.08 atm lit. I mol/K = 2 Cal/K/mol J]
Find the work done when 2 moles of hydrogen expand isothermally from 15 to 50 litres against a constant pressure of 1 atm at 25°C.
An ideal gas expands in volume from 1 × 10−3 to 1 × 10−2 m3 at 300 K against a constant pressure of 1 × 105 Nm−2. The work done is ______.
