Advertisements
Advertisements
प्रश्न
A body is performing S.H.M. Then its ______.
- average total energy per cycle is equal to its maximum kinetic energy.
- average kinetic energy per cycle is equal to half of its maximum kinetic energy.
- mean velocity over a complete cycle is equal to `2/π` times of its π maximum velocity.
- root mean square velocity is times of its maximum velocity `1/sqrt(2)`.
उत्तर
a, b and d
Explanation:
In the case of S.H.M, the average total energy per cycle
= Maximum kinetic energy (K0)
= Maximum potential energy (U0)
Average KE per cycle = `(0 + K_0)/2 = K_0/2`
Let us write the equation for the SHM x = a sin ωt.
Clearly, it is a periodic motion as it involves a sine function.
Let us find velocity of the particle, `v = (dx)/(dt) = d/(dt) (a sin ωt) = aω cos ωt`
Mean velocity over a complete cycle,
`v_"mean" = (int_0^(2pi) ωa cos θd θ)/(2pi)`
= `(ωa[sin θ]_0^(2pi))/(2pi)`
= 0
So, `v_"mean" ≠ 2/pi v_"max"`
Root mean square speed,
`v_(rms) = sqrt((v_"min"^2 + v_"max"^2)/2`
= `sqrt((0 + v_"max"^2)/2`
`v_(rms) = 1/sqrt(2) v_"max"`
APPEARS IN
संबंधित प्रश्न
The maximum speed and acceleration of a particle executing simple harmonic motion are 10 cm/s and 50 cm/s2. Find the position(s) of the particle when the speed is 8 cm/s.
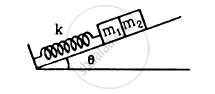
The block of mass m1 shown in figure is fastened to the spring and the block of mass m2 is placed against it. (a) Find the compression of the spring in the equilibrium position. (b) The blocks are pushed a further distance (2/k) (m1 + m2)g sin θ against the spring and released. Find the position where the two blocks separate. (c) What is the common speed of blocks at the time of separation?
Find the elastic potential energy stored in each spring shown in figure, when the block is in equilibrium. Also find the time period of vertical oscillation of the block.
Solve the previous problem if the pulley has a moment of inertia I about its axis and the string does not slip over it.
A 1 kg block is executing simple harmonic motion of amplitude 0.1 m on a smooth horizontal surface under the restoring force of a spring of spring constant 100 N/m. A block of mass 3 kg is gently placed on it at the instant it passes through the mean position. Assuming that the two blocks move together, find the frequency and the amplitude of the motion.
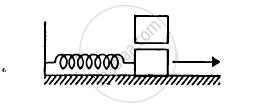
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
Displacement versus time curve for a particle executing S.H.M. is shown in figure. Identify the points marked at which (i) velocity of the oscillator is zero, (ii) speed of the oscillator is maximum.

Find the displacement of a simple harmonic oscillator at which its P.E. is half of the maximum energy of the oscillator.
